Spatial and temporal analysis of extreme storm-tide and skew-surge events around the coastline of New Zealand

Coastal flooding is a major global hazard, yet few studies have examined the spatial and temporal characteristics of extreme sea level and associated coastal flooding. Here we analyse sea-level records around the coast of New Zealand (NZ) to quantify extreme storm-tide and skew-surge frequency and magnitude. We identify the relative magnitude of sea-level components contributing to 85 extreme sea level and 135 extreme skew-surge events recorded in NZ since 1900. We then examine the spatial and temporal clustering of these extreme storm-tide and skew-surge events and identify typical storm tracks and weather types associated with the spatial clusters of extreme events. We find that most extreme storm tides were driven by moderate skew surges combined with high perigean spring tides. The spring–neap tidal cycle, coupled with a moderate surge climatology, prevents successive extreme storm-tide events from happening within 4–10 d of each other, and generally there are at least 10 d between extreme storm-tide events. This is similar to findings from the UK (Haigh et al., 2016), despite NZ having smaller tides. Extreme events more commonly impacted the east coast of the North Island of NZ during blocking weather types, and the South Island and west coast of the North Island during trough weather types. The seasonal distribution of both extreme storm-tide and skew-surge events closely follows the seasonal pattern of mean sea-level anomaly (MSLA) – MSLA was positive in 92 % of all extreme storm-tide events and in 88 % of all extreme skew-surge events. The strong influence of low-amplitude (−0.06 to 0.28 m) MSLA on the timing of extreme events shows that mean sea-level rise (SLR) of similarly small height will drive rapid increases in the frequency of presently rare extreme sea levels. These findings have important implications for flood management, emergency response and the insurance sector, because impacts and losses may be correlated in space and time.

1 Introduction

Coastal flooding is a major global hazard with historical events killing hundreds of thousands of people and causing billions of dollars in damage to property and infrastructure (e.g. Lagmay et al., 2015; Needham et al., 2015; Haigh et al., 2016). Globally, it has been estimated that up to 310 million people are already exposed to a 1 in 100-year flood from the sea (Jongman et al., 2012; Hinkel et al., 2014; Muis et al., 2016). In New Zealand this includes 72 000 people – 1.5 % of the population (Paulik et al., 2019, 2020). This will get worse, since without adaptation, it has been estimated that 0.2 %–4.6 % of global population will be flooded annually under 25–123 cm (RCP2.6–RCP8.5) of global mean sea-level rise (SLR; Hinkel et al., 2014). Improved understanding of extreme sea level and coastal flooding events is therefore important.

To understand and manage their risk exposure, central government agencies, environmental and emergency managers and the insurance and financial sectors, all require knowledge of the likely frequency and magnitude of extreme storm-tide events and their clustering in time and space. Studies have quantified the frequency and magnitude of extreme sea levels at regional (e.g. Bernier and Thompson, 2006; I. Haigh et al., 2010; I. D. Haigh et al., 2010, 2014) and global (e.g. Menéndez and Woodworth, 2010; Muis et al., 2016) scales, but, other than Haigh et al. (2016), recognition and analysis of spatial and temporal extreme sea-level characteristics and associated coastal flooding is lacking globally. Haigh et al. (2016) provided an analysis of spatial and temporal extreme sea-level (storm-tide) characteristics for the UK coastline. In this paper we undertake a similar analysis for the New Zealand (NZ) coastline, contributing to a growing understanding of spatial and temporal extreme sea-level characteristics worldwide for different tidal and storm contexts.

In the past, relatively few NZ sea-level records have been included in global extreme sea-level studies (e.g. Wahl et al., 2017) whose focus was on global intra-comparisons and trends. Therefore, here we provide a comprehensive assessment of extreme sea level and skew surge in NZ based on a more extensive tide gauge dataset (with on-average records of 30 years in length). We use sea-level gauge records to quantify the frequency–magnitude distribution of extreme sea level and skew surges. Skew surge is the height difference between a sea-level (storm-tide) peak and the nearest high tide. We also examine the influence of the mean sea-level anomaly (MSLA) on the timing of extreme storm-tide events. MSLA is derived from the low-frequency non-tidal residual sea level (Merrifield et al., 2013), which is dominated by seasonal heating and cooling of the sea (Bell and Goring, 1998; Boon, 2013) and inter-annual climate cycles such as El Niño–Southern Oscillation (Goring and Bell, 1999) and is often not considered in extreme storm-tide analyses because in most places it is small compared to tide and skew surge – we show that it has an important influence on the timing of extreme storm-tide and skew-surge events in NZ. We analyse the spatial and temporal clustering of extreme storm-tide and skew-surge events and identify typical storm tracks and weather types associated with spatial clusters of extreme events.

2 New Zealand storm-tide characteristics

Tsunami and land subsidence aside, there are several meteorological and astronomical influences on sea level that can combine in a number of ways to inundate low-lying coastal margins: the height of mean sea level (MSL) relative to a local datum or landmark, astronomical tides (referred to henceforth simply as tide), synoptic weather-induced storm surge, MSLA (caused by seasonal, interannual and inter-decadal climate variability), long-term change in MSL through SLR, and wave set-up and run-up. We use the term MSLA in recognition that the anomaly is calculated relative to MSL, after MSL and any long-term MSL trend has been removed (described later). It is common to treat the wave-induced set-up and run-up separately from the still (non-wave) water level because sea-level gauges are usually placed inside harbours to minimize the effects of wave set-up and run-up. Here we define the term “storm tide” as the total water level above MSL from the sum of tide plus storm surge plus MSLA. Since skew surge is the height difference between a storm-tide peak and the nearest predicted high tide, then skew surge contains a component of both storm surge plus MSLA.

NZ tides are mostly meso-tidal (2–4 m tidal range) but with some micro-tidal (< 2 m range) locations (e.g. Wellington). The tidal regime is mixed semi-diurnal, experiencing two high tides per day of which one high tide is larger than the other. Tides are larger on the west coast where the semi-diurnal signal is stronger and there is a strong fortnightly spring–neap cycle. Tides are smaller on the east coast where an amphidrome in the diurnal S₂ constituent leads to a more noticeable monthly perigean influence in the peak tides (Walters et al., 2001). Tides flow west to east through the constricted Cook Strait (which separates the North Island and the South Island, Fig. 1), and the constriction causes the largest tides to occur near Nelson (site 22, Fig. 1) and the smallest tides at Wellington (site 11, Fig. 1).

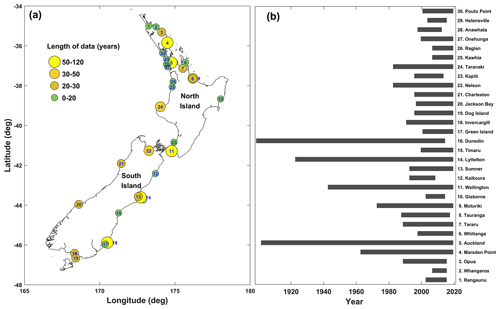
Figure 1 (a) Location of tide gauge sites around NZ, with site number and (b) duration of the sea-level records.

NZ does not experience tropical cyclones (hurricanes) and has a relatively deep and narrow continental shelf and so does not experience very large storm surges on a global scale – the semi-diurnal tides dominate sea-level variability and storm surges are limited to mostly < 0.5 m, which is approximately 25 % of the average tidal range (Stephens et al., 2014). Rueda et al. (2017) classify NZ's coastal flood hazard climate as being “lightly unbounded” (extreme storm-tide shape parameter weakly positive), “macro-level” (total water level ≥1 m), “highly variable” (high interannual variability) and “tide-dominant” (tidal range dominates the total water level height compared to storm surge and wave set-up).

3 Data and methods

We analysed sea-level records from 30 locations in NZ (Fig. 1). The duration of the records ranged from 6 to 115 years with a mean and median of 31 and 20 years respectively, with mostly concurrent records since the early 1990s. The sea-level records were quality-analysed to remove any spikes, timing errors, or datum shifts but leaving any data gaps. Tide gauge stations globally are typically located in protected embayments, which limits the direct impact of wind waves relative to open coastlines, but storm surges (and skew surges) generally are well sampled by tide gauges (e.g. Merrifield et al., 2013), provided no seiching occurs, or, if it does occur, the sampling rate is fine enough to resolve the seiche. The NZ sea-level records analysed here are from a variety of locations including wave-exposed open coast, inside port breakwaters, or mounted on wharves inside estuaries, so they have different levels of wave exposure. The sampling frequency varies between and within individual records, from 1 min up to 1 h sampling intervals. At some sites far infragravity waves of > 2 min and several decimetres amplitude are observed in the 1 min data during local or remote storm events (Thiebaut et al., 2013), and tsunami events are occasionally recorded too. To enable consistent analysis between gauges and within long-duration records with varied sampling frequency, we subsampled all sea-level data to 1 h intervals after first applying a 15 min running average to minimize the effect of far infragravity and tsunami waves. Thus, we analyse still-water levels with short-period wave effects minimized. Some open-coast sites can experience wave set-up when large waves are present.

The sea-level heights are specified relative to local vertical datum (Hannah and Bell, 2012). Average relative mean sea levels in New Zealand have exhibited an approximately linear rise over the last century of 1.7±0.1 mm yr⁻¹ (Hannah and Bell, 2012). Therefore, before further analysis the data were linearly detrended to a zero mean relative to local vertical datum for each gauge, to remove the effects of historical SLR from the sea-level distribution and create a quasi-stationary time series required for extreme value analysis (Coles, 2001). We used a linear trend rather than removing annual mean sea level because we wished to retain inter-annual sea-level variability as a long-period component of MSLA in the storm-tide distribution.

The detrended sea-level records were separated into their main component parts. Tidal elevations were predicted using harmonic analysis with 67 constituents following Foreman et al. (2009), and the tides were subtracted from the detrended sea level to obtain the non-tidal residual. The solar annual and semi-annual tidal constituents were omitted from the tidal harmonic predictions because most of the seasonal signal is actually driven by non-astronomical effects like seasonal heating and cooling (Bell and Goring, 1998; Boon, 2013), and we wished to later analyse seasonal effects on the timing of extreme storm-tide events. MSLA was calculated using a 30 d running average of the non-tidal residual (Haigh et al., 2014) – MSLA is a slowly varying (≥1 month) component of both storm tide and skew surge, which includes seasonal sea-level variability. Skew surge was calculated as the absolute difference between the maximum recorded storm tide during each tidal cycle and the predicted maximum astronomical tidal level for that cycle, irrespective of differences in timing between these (Batstone et al., 2013; Williams et al., 2016) – every high tide has an associated skew surge. Skew surge is a relevant metric of surge in tidally dominant locations like NZ (e.g. Merrifield et al., 2013) because the extreme storm tide and resulting flooding exposure (excluding wave overtopping) usually occurs for a few hours around the high tide.

Like Haigh et al. (2016), we investigate two types of events: (1) extreme storm-tide events (relevant to coastal flooding) that reached or exceeded the 1 in 5-year return level (the storm tide equalled or exceeded once, on average, every 5 years) in the detrended series (i.e. independent of SLR) and (2) extreme skew surges that reached or exceeded the 1 in 5-year return level. Some of the skew-surge events coincide with the extreme storm-tide events, when the storm surge occurred around the time of high water of a spring tide; others do not coincide, because the surge occurred near a small high tide or on a neap tide. In this paper an “event” is defined as a meteorological storm that caused an extreme storm tide or skew surge at least one tide gauge but often at several tide gauges – so a single “event” can have multiple observed extremes.

A generalized Pareto distribution (GPD) fitted to peaks-over-threshold (POT) data (Coles, 2001) was used to determine the 1 in 5-year return level thresholds for identifying extreme storm-tide and skew-surge events, because the GPD model is fitted directly to the independent storm-tide and skew-surge maxima (e.g. Fig. S1 in the Supplement) associated with storms that we analyse later. POT consisted of sea-level maxima with the maxima being separated by a minimum of 3 d, since separate meteorological systems generally pass over NZ within 4–7 d of each other. A test using a 2 d threshold for both storm tide and skew surge showed that the identification of extreme events was insensitive to the time threshold. The POT height threshold was selected to give an average of approximately five high-water maxima per year over the duration of the measurement record, which is equivalent to about the 99.8th percentile of the hourly data (the number of maxima exceeding the height threshold varies year to year). However, for predicting long return-period levels such as for 100 years, the GPD model is likely to be biased low when analysing short sea-level records (e.g. Fig. S1) due to the likelihood of observing few very large maxima over a short record (e.g. I. D. Haigh et al., 2010). Therefore, we also applied the skew-surge joint-probability method (SSJPM) (Batstone et al., 2013) to determine extreme storm-tide frequency and magnitude. Joint-probability methods provide more robust low-frequency magnitude estimates for short-duration records because they overcome the main theoretical limitations of extreme value theory application to sea levels – splitting the sea level into its deterministic (predictable) tidal and stochastic (e.g. unpredictable, storm-driven) non-tidal components, and analysing the two components separately before recombining (e.g. Tawn and Vassie, 1989; I. D. Haigh et al., 2010). Storm-tide return periods can be estimated from relatively short records because all skew surges are considered, not just those that lead to extreme levels. A limitation of the SSJPM and other joint-probability methods is that it assumes tide and skew surge are independent, which has been shown to be true in the UK (Williams et al., 2016) but has not been fully investigated in NZ, although comparisons with direct maxima methods for > 50-year-long records (Fig. S1) give similar results for return periods ≥10 years and also match observed maxima well, and thus support the validity of the independence assumption for long-return-period events. Santamaria-Aguilar and Vafeidis (2018) found skew surge to be independent of high-tide height in mixed semi-diurnal tidal regimes located in regions with a narrow continental shelf, like NZ. However, this may not be the case inside harbours where storm surge magnitude can depend on the tidal stage (e.g. Bernier and Thompson 2007; Horsburgh and Wilson, 2007; Goring et al., 2011). In summary, the GPD/POT model was used to determine extreme skew-surge frequency and magnitude and to identify extreme storm tides and skew surges with ≥1 in 5-year return levels for further analysis. We used the SSJPM to determine the return period of extreme storm tides (e.g. Fig. 2a, Supplement Tables S2, S4 and S7) when the GPD and SSJPM methods both indicated a ≥10-year return period for a particular storm tide, since we prefer the SSJPM method but there can be a mismatch between the GPD and SSJPM at the 5-year return level used for storm-tide maxima selection (Fig. S1). Using a SSJPM-based storm-tide threshold would select a slightly different set of storm tides for analysis but would not affect the key results or conclusions.

To characterize storms in the wider NZ region, we used the Kidson (2000) classification of synoptic weather regimes associated with clusters of extreme storm tides and/or skew surges that impacted at least three tide gauge sites during the event. Kidson (2000) defined three weather “regimes”, characterized by (i) frequent low-pressure troughs crossing the country, (ii) high-pressure systems to the north with strong zonal flow to the south of the NZ, and (iii) blocking patterns with high-pressure systems more prominent in the south (Fig. S2). Rueda et al. (2019) used a method of weather typing to develop statistical predictors for storm surge and wave height in NZ based on statistical relationship with MSL pressure fields. The Kidson (2000) weather regimes are easily associated with the extreme storm-tide and skew-surge spatial clusters identified.

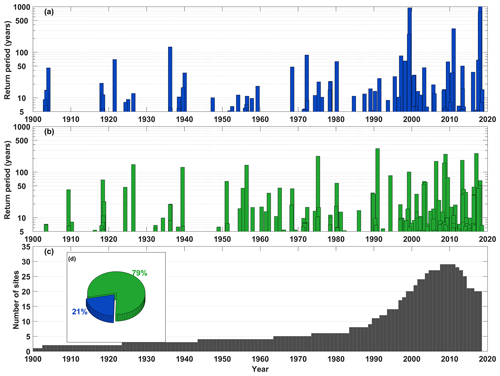
Figure 2 (a) Return period of the highest storm tides in each of the 85 extreme storm-tide events (resulting in 155 extreme storm-tide observations), offset for mean sea level; (b) return period of the highest skew surges in each of the 135 skew-surge events (resulting in 191 extreme skew-surge observations); (c) the number of sites per annum for which sea-level data are available across the 30 sites; and (d) pie chart showing the number of skew-surge events that led to extreme storm-tide events (blue) and the number that did not (green).

4 Results

4.1 Comparison of events

We compared the dates and return periods for both extreme storm-tide and extreme skew-surge events and ascertained how many skew-surge events also resulted in extreme storm tides. Extreme storm tides were generated by 85 distinct storm events (Fig. 2a, Table S2). These 85 storm events contributed to 155 independent storm-tide maxima that reached or exceeded the 1 in 5-year return level at any of the 30 sea-level-gauge sites (Fig. 1) – in other words the storm events sometimes caused extreme storm tides to occur at more than one sea-level gauge site. In total, 191 skew surges reached or exceeded the 1 in 5-year return period across the 30 sites, generated by 135 distinct storm events (Fig. 2b, Table S3). This difference in event numbers is consistent with NZ's coastal context of meso- to micro-tidal ranges and moderate storm surges, which are usually several decimetres (not metres) high, meaning tides are a key determinant (or precursor) for coastal flooding rather than solely storm surges.

Like Haigh et al. (2016), there are unavoidable issues with the database that arise because hourly tide gauge records do not cover all the full 118-year period analysed since the start of the Auckland and Dunedin records in 1900. It is obvious, examining Fig. 2c, that we are likely missing many events before the early 1990s, when records were spatially sparse. The decline in data availability post-2010 occurs due to discontinuation of some sea-level gauges around that time, but there are also some recent records that we were unable to obtain for analysis. The highest return period from two notable historical storms is probably lower than it should be, e.g. the great 1936 ex-tropical cyclone (Brenstrum, 2000) and ex-tropical cyclone Giselle in 1968 (Revell and Gorman, 2003). Although we have data at some sites for these events, tide gauges were not necessarily operational at the time along the stretches of the coastline where the storm tides or skew surges were likely to have been most extreme; this is the case for the 1936 (except in Auckland) and 1968 events. Notwithstanding these issues, our analysis of the events that we have on record does provide important insights, as described below.

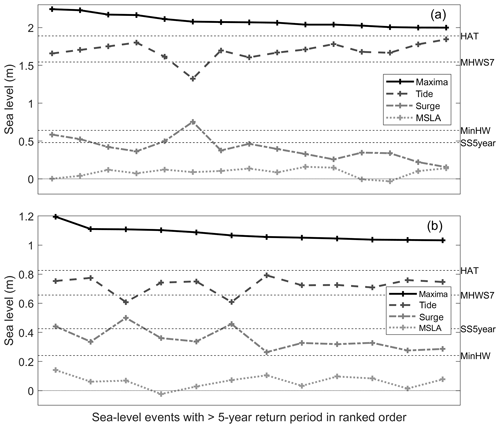
Figure 3 The contributions of tide, skew surge and MSLA to the ≥1 in 5-year return period extreme storm-tide maxima at two of NZ's longest sea-level recorders with a meso-tidal (a Auckland) and micro-tidal (b Wellington) range. HAT denotes the highest astronomical tide, MHWS-7 is the height equalled or exceeded by the highest 7 % of all high tides, MinHW denotes lowest high tide height; SS5year is the height of the 1 in 5-year return period skew surge.

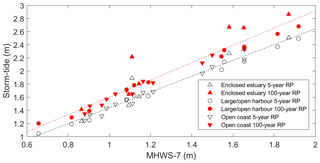
It is not possible for tide alone to result in a ≥1 in 5-year return period extreme storm tide, so all observed storm tides were associated with storms that produced skew surge. However, only 29 of the 135 (21 %) extreme skew-surge events led to extreme storm tides (Fig. 2d), while the majority (79 %) did not. Hence, as Haigh et al. (2016) found for the UK coast, most extreme storm tides arose from moderate (i.e. < 1 in 5-year return levels) skew-surge events combined with astronomical spring high tides. Occasionally a smaller high tide combined with a large skew surge to produce an extreme storm tide – more so for sites with a micro-tidal range (e.g. Wellington; see Fig. 3). The ratio of maximum observed skew surge to maximum observed storm tide ranged from 0.23 to 0.51 with a median of 0.34 (Fig. S3). The tide is usually the dominant component of sea-level height, even during extreme events, and extreme storm tides occur close to high tide and are unlikely during a small neap high tide. Extreme storm-tide elevation is linearly related to mean high water spring (MHWS) elevation (Fig. 4). Equations 1 and 2 are the least-squares linear fits between the MHWS-7 (the height equalled or exceeded by the highest 7 % of all high tides) and the 5-year and 100-year return storm tide, respectively. Outliers to the linear fits occur in some upper-estuarine locations where the skew-surge / storm-tide ratios are relatively large (Fig. S3).

$$\begin{array}{}\text{(1)}& {\displaystyle }\begin{array}{rl}& \text{5-year return period extreme storm tide (m)}\\ & =\mathrm{1.20}\times \text{MHWS-7}+\mathrm{0.23}\end{array}\text{(2)}& {\displaystyle }\begin{array}{rl}& \text{100-year return period extreme storm tide (m)}\\ & =\mathrm{1.32}\times \text{MHWS-7}+\mathrm{0.28}\end{array}\end{array}$$

During the highest extreme storm tides, all sea-level components tended to be large and positive (Fig. S4), with the tide being the largest component, but skew surge and MSLA being relatively larger in the highest storm tides (Fig. S5). The MSLA was positive in 92 % of all extreme storm tides and in 88 % of all extreme skew surges.

Figure 4 Linear relationship between high tide and storm tide in NZ. MHWS-7 is the height equalled or exceeded by the highest 7 % of all high tides. RP denotes return period, also known as average recurrence interval.

4.2 Spatial analysis

We then considered the spatial characteristics of events around the coast. As expected, and as Haigh et al. (2016) noted for the UK, there is a significant (95 % confidence level) correlation (0.50 for the storm-tide events and 0.47 for the skew-surge events) between the highest return period of the events and the number of tide gauge sites impacted (Fig. S6).

To investigate the different spatial extents of coastline affected, we identified 30 storm events that impacted three or more gauge sites concurrently (Fig. 5). We observed two separate categories of spatial footprints, which can be related to the Kidson (2000) weather regimes (Fig. S2) as follows:

1. Category 1 footprints predominantly impacted the northeast coast of the North Island and occasionally the west coast of the North Island (see Fig. 1a). Category 1 footprints were most commonly associated with blocking weather types (Fig. 5), low-pressure systems that tracked from north of NZ (Fig. 6) and intensified next to a blocking high-pressure system lying east of NZ. Category 1 footprints had storm centres that lay over the North Island or north of NZ at the time of maximum storm tide or skew surge, with a mean position located just off the northeast coast of the North Island (Fig. 6).

2. Category 2 footprints impacted everywhere but mainly the South Island (Fig. 1a) and the west coast of the North Island (Fig. 5). Category 2 footprints were generally associated with trough weather regimes, which were the most common event drivers. Troughs were associated with storm centres that tracked eastwards across NZ, usually across or south of the South Island and always south of the northernmost tip of NZ (Fig. 6). Category 2 footprints had storm centres at the time of maximum storm tide that lay in an arc across the South Island from northwest to southeast, with a mean position located southeast of the South Island (Fig. 6).

The largest events with return periods ≥1 in 50 years were more common on the east coast of the North Island during blocking weather types, whereas trough weather types were more common in the South Island and along the west coast of the North Island (Fig. 5).

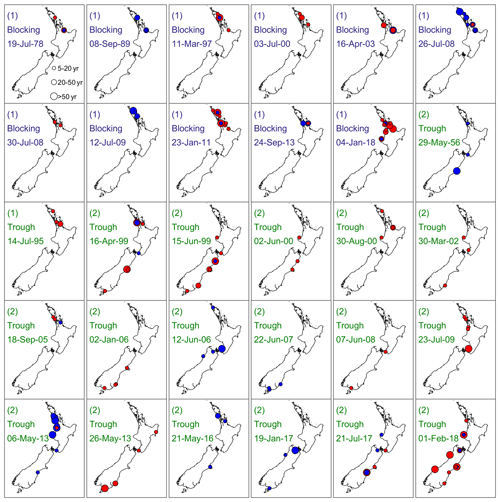
Figure 5 The spatial footprints of all the storm-tide or skew-surge events that impacted at least three tide gauge sites. Red circle denotes extreme storm tide; blue circle denotes extreme skew surge. Circle diameter matched to return periods of 5–20, 20–50 and ≥50 years (small to large respectively). The spatial cluster category (1 or 2), Kidson weather type and date during each event are shown.

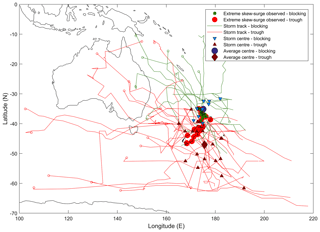
Figure 6 Storm tracks and weather types contributing to all the storm-tide or skew-surge events that impacted at least three tide gauge sites. Storm tracks and the location of the storm centre at storm-tide event peak are marked for both blocking (green and blue) and trough (red and brown) Kidson (2000) weather types. Open circles denote storm track origin.

4.3 Temporal analysis

Next, we examined the temporal variation in events. Extreme storm-tide events with ≥1 in 5-year return level occur throughout the year but are least frequent in late winter and early spring (September–December) (Fig. 7). Extreme storm-tide events with ≥1 in 25-year return level exhibit a peak in January that tapers off through to May followed by another peak in June that tapers off through to September. No storm-tide events with ≥1 in 25-year return level were observed in September–December. Like extreme storm tide, skew surges with ≥1 in 5-year return level are also least likely in the latter half of the year and were most frequent March–July.

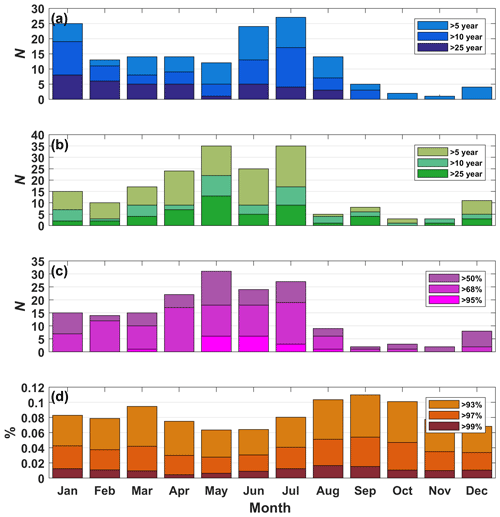
Figure 7 Seasonal distribution of sea-level components associated with extreme storm-tide and skew-surge events: (a) extreme storm tide, (b) extreme skew surge, (c) MSLA and (d) high tide ≥93rd percentile (includes all high tides, not just those associated with extreme storm-tide events). N denotes number of occasions.

The largest tidal constituents around NZ are the M2, S2 and N2 semi-diurnal constituents. The seasonal pattern in the height of the highest tides, which peak around March and September (Fig. 7d), is affected by the solar equinox. However, the seasonal pattern in the number of extreme storm-tide and skew-surge events does not follow the highest tides but appears to follow the seasonal pattern of MSLA – MSLA being a component of both extreme storm-tide and skew-surge elevations. The mean annual MSLA cycle is dominated by thermo-steric sea-level adjustments and secondary-forcing variables of barometric pressure and alongshore wind stress (Bell and Goring, 1998), and it peaks between March and June in NZ – at 22 of 30 sites it peaks in May due to the thermosteric lag. The range of the 95 % confidence intervals of the annual sea-level cycle was about −0.15 to +0.15 m around NZ, and the median amplitude across all sites was 0.04 m (Fig. S7). Yet MSLA can be larger in any single month and tended to be larger during observed extreme events – MSLA during events ranged from −0.06 to 0.28 m (Tables S2 and S3). The MSLA influence on the timing of extreme storm tide is perhaps surprising given the dominance of tide on the storm-tide elevation (Figs. 3 and 4). But because two spring tidal cycles per month provide sufficient monthly “exposure potential” for storm-tide events, the seasonal pattern is then strongly influenced by the mean annual MSLA cycle. Once the effect of the mean annual MSLA cycle is removed, the seasonal distributions of both extreme storm-tide and skew-surge events are more uniform throughout the year (Figs. S8 and S9), although there is still a tendency for a lower number of extreme storm-tide and skew-surge events near the end of the year in October–December.

In terms of inter-annual climate effects on extreme storm tides, we found no relationship between extreme storm-tide height or return period and large-scale climate modes, such as the El Niño–Southern Oscillation (ENSO) and Southern Annular Mode (SAM; e.g. Fig. S10, Table S10 Trenberth, 1976). This aligns with the findings of Lorrey et al. (2014) that ex-tropical cyclone tracks and locations relative to Auckland at their point of closest passage are not directly linked to the phase of ENSO or SAM, but they are linked more closely to atmospheric circulation conditions that develop in the southwestern Pacific, to the north of and over New Zealand. In other words, extreme storm-tide events are influenced by random weather events rather than large-scale climate variability modes.

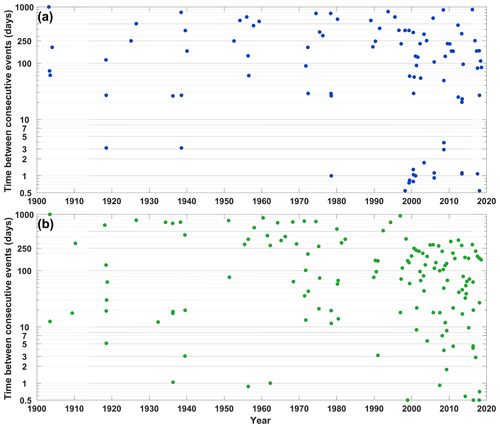
Following Haigh et al. (2016), we examined temporal clustering by considering the number of days between consecutive events. There are no instances of storm-tide events happening within 4–10 d of each other, whereas there are occasions when pairs of skew-surge events occurred within that time interval (Fig. 8). Only once did two extreme storm-tide events occur within < 4 d of each other, when two separate low-pressure systems intensified against a blocking high-pressure system and impacted NZ on 27 and 30 July 2008.

Figure 8 Time between successive events: (a) storm tide and (b) skew surge.

5 Discussion

5.1 Tidal influence

In NZ, we find that most of the extreme storm tides are driven by moderate skew surges combined with high spring or perigean spring tides, which is similar to the UK despite the fact that the UK has larger tides and a slightly smaller surge / tide ratio (Figs. S3c and S10c of Haigh et al., 2016) and is also similar for annual maxima around much of the world (Merrifield et al., 2013). We found that extreme storm-tide elevations are strongly correlated with MHWS elevation. This affirms current practice in NZ of forecasting “red-alert” tide dates when high-tide peaks are predicted to be unusually high (Bell, 2010; Stephens et al., 2014) (see NZ Storm-Tide Red-Alert Days; National Institute of Water and Atmospheric Research; https://niwa.co.nz/our-science/coasts/tools-and-resources/tide-resources, last access: 10 March 2020). The red-alert tide concept works in New Zealand because the semi-diurnal tides dominate sea-level variability and storm surges are limited to mostly < 0.5 m, which is approximately 25 % of the average tidal range (Stephens et al., 2014). Coastal or hazard managers are advised to keep a close watch on the weather for lower barometric pressure and adverse winds during the red-alert tide days, as even a minor storm or swell event could lead to inundation of low-lying areas, especially if accompanied by waves (Bell, 2010). In practice, weather forecasts alert managers to high surges and storm tides with < 10 d notice, but the red-alert tide calendars enable resource planning (e.g. staff leave days) months in advance of potential high-storm-tide days and are a valued coastal climate service in NZ. For example, the highest storm tide in Auckland (NZ) on record since 1900 occurred on 23 January 2011, closing major city highways, and causing millions of dollars of flood-related damage. At 0.52 m the skew surge was relatively large for NZ and coincided with a predicted “red alert” (high perigean spring) tide, and a background MSLA of nearly 0.05 m, noting that ongoing SLR increasingly adds to the impact or frequency of flooding (Stephens et al., 2018). SLR of about 0.12 m between 1936 and 2011 means that after detrending for sea-level rise the 2011 storm tide is actually the second-largest storm tide with the 26 March 1936 storm tide being largest (Fig. 3a).

The observed linear relationships between extreme storm tide and MHWS elevation have proven useful for estimation of extreme storm tide at locations without long-term sea-level gauges – extreme storm tide can be predicted using short-term sea-level recorder deployments to establish tidal elevations, or a tidal model (e.g. see New Zealand Tide Forecaster; https://niwa.co.nz/our-science/coasts/tools-and-resources/tide-resources). However, relatively large extreme storm tide in some upper-estuarine locations plotted as outliers to the linear relationships (Figs. 4, S3). Research has shown that non-linear interactions between tide, wind and morphology influence surge generation inside enclosed estuaries (e.g. Plüß et al., 2001; Rego and Li, 2010; Orton et al., 2012), a subject for further research in NZ since there are few long-term sea-level records to help quantify extreme storm-tide frequency and magnitude in NZ upper-estuarine locations.

5.2 MSLA influence

The median amplitude of the mean annual sea-level cycle from all sea-level records is only 0.04 m, yet the annual MSLA cycle is a strong influence on the monthly timing of extreme storm-tide and skew-surge events. MSLA was positive in 92 % of all sea level and in 88 % of all extreme skew-surge events and is clearly an influential component of the highest storm tides (Figs. S3–S4, S7–S8). The sensitivity of extreme storm tide to relatively small MSLA shows that small increases in mean sea level, from long-term SLR, will cause a large increase in the frequency of extreme storm tide, a result consistent with other studies (e.g. Hunter, 2012; Sweet and Park, 2014; Stephens et al., 2018). Tidally dominated sites like NZ, where the tide is the key determinant of extreme storm tide, will be more sensitive to SLR in terms of the increasing frequency of extreme storm-tide events than surge-dominated sites (Vitousek et al., 2017; Rueda et al., 2017; Stephens et al., 2018). This is because tidally dominated sites have a relatively flat upper tail on the extreme storm-tide frequency–magnitude distribution. Many parts of the world are even more tidally dominant than NZ (Merrifield et al., 2013; Rueda et al., 2017) and will experience earlier emergence of flooding due to greater sensitivity to SLR (Hunter, 2012).

5.3 Surge influence

NZ is similar to the UK in that temporal clustering of storm-tide events is controlled by the spring tide cycle (Haigh et al., 2016), with storm-tide events generally not occurring within 10 d of each other historically. However, it only requires storm surge to elevate high tides to an extreme storm-tide level. Therefore, extreme storm tides and skew surges do not strike NZ-wide but exhibit spatial clustering associated with storm tracks that have general patterns related to weather types.

During the spring and summer of 2017 and 2018 several large storms including ex-tropical cyclones Fehi, Gita and Hola struck NZ, most of them coinciding with high perigean spring tides, causing flooding to homes and damaging infrastructure. Other notable historical coastal flooding events in NZ occurred in January 2011, during cyclone Giselle in 1968 (de Lange and Gibb, 2000), May 1938 in the Hauraki Plains (Stephens, 2018) and during the great cyclone of 1936 (Brenstrum, 2000), but the spatial effects of these historical storms are not well recorded since many sea-level gauges were not operating at those times (Fig. 1). The calculated extreme storm-tide and skew-surge frequency and magnitudes (Tables S7 and S8) are based on available digital records but could be biased low in places where historical storms are not included. A notable example is in Tauranga Harbour, where the highest skew surge ever recorded in NZ by a sea-level gauge occurred during cyclone Giselle in 1968 and reached 0.88 m above the predicted high tide (de Lange and Gibb, 2000). However, that tide gauge is no longer operating and hourly data were not available for inclusion in this analysis. We applied a joint-probability method to determine the frequency–magnitude distribution of extreme storm tide (Table S7), which partially overcomes the limitation of short-duration sea-level records.

Before the analysis presented here, the largest recorded skew surge was 0.88 m measured in Tauranga Harbour in 1968. We observed 15 larger skew surges at enclosed estuarine sites (Fig. 1a): 1 at Helensville (site 29), 1 at Raglan (site 26), 2 at Kawhia (site 25) and 11 at Invercargill (site 18). The maximum skew surge from our analysis was 1.15 m at Raglan on 6 May 2013, and a skew surge of 1.26 m was inferred from anecdotal evidence in the northern Hauraki Plains on 4 May 1938 (Table S2, Stephens, 2018). However, a much larger non-tidal residual sea level (NTR) was measured at the open-coast Jackson Bay site around low tide, during ex-tropical cyclone Fehi on 1 February 2018 (Fig. S12). NTR is the difference between the measured storm tide and the predicted tide and is calculated over the full tidal cycle at the sampling frequency of the sea-level record, hourly in this case. The NTR at Jackson Bay on 1 February 2018 appeared to be a meteorologically forced wave that peaked at 2.29 m near low tide (Fig. S12) and would have created a much higher storm tide if it had peaked at high tide – the skew surge was 0.69 m. Although this was the highest storm tide on record at Jackson Bay, it was a near miss to a much higher storm tide. We found that the “potential” storm tide, constructed from the sum of the maximum observed tide and skew surge, was 7 %–20 % higher than the estimated 100-year return period extreme storm-tide level (Table S9). Hence the potential exists for higher and more damaging storm tides than historically observed in the patchy NZ records, even without considering the effects of future SLR. Alongside the provided frequency–magnitude estimates, it would be sensible to consider these “potential” extreme storm-tide elevations when planning or managing low-lying coastal land use.

We analysed still-water levels with wave effects removed from the record (apart from wave set-up that may be implicit in measurements for some events at open-coast gauges, e.g. site 8). Waves are often present during storms, and wave set-up and run-up can raise the water level at the coast substantially, especially on steeper beach gradients or steep-face structures such as rock revetments or seawalls (e.g. Stockdon et al., 2006; Stephens et al., 2011). Hazard analyses should account for wave effects, including far infragravity surges, in wave-exposed locations, on top of the extreme storm tide presented here. An analysis of the spatial and temporal clustering of extreme wave events could also be undertaken, following the approach of Santos et al. (2017).

We analysed extreme storm tide and skew surge for 30 sea-level records of varying length throughout NZ. Numerical models, properly calibrated, provide a means of extending the extreme storm-tide analyses to develop probabilistic assessments of total water level along the entire coastline. These can include combinations of tidal (e.g. Walters et al., 2001), storm-surge and wave models (e.g. Gorman et al., 2003; Rueda et al., 2019), which can include both hindcasts and climate-change future casts (Cagigal et al., 2019). However, numerical storm-surge models for climate change typically do not include MSLA, which is an important component of extreme storm tide (for tidally dominant coasts) and hence flooding exposure (e.g. Stephens et al., 2014; Sweet and Park, 2014), and which we have shown to be an important influence on the timing of extreme events. Efforts to forecast MSLA are being made (Widlansky et al., 2017), but work is required to include MSLA into models for the development of probabilistic assessment of extreme water levels to assess coastal hazard risks, taking into account SLR.

6 Conclusions

In this paper we analysed sea-level records to quantify extreme storm-tide and skew-surge frequency and magnitude around the coast of NZ for the first time. We identified the relative magnitude of sea-level components contributing to 85 extreme storm-tide and 135 extreme skew-surge events (≥5-year return period), which have been recorded in NZ since 1900. We examined spatial and temporal clustering of extreme storm-tide and skew-surge events and identified typical storm-tracks and weather types in the SW Pacific associated with spatial clusters of extreme sea levels.

We found that around NZ most extreme storm tides are driven by moderate skew surges combined with high perigean spring tides. Extreme storm-tide elevations are highly correlated with and linearly related to mean spring high-tide elevation, enabling prediction of extreme storm-tide elevations in ungauged areas from tidal information, which is readily available from short-term measurements or tidal models.

The spring–neap tidal cycle, coupled with a small to moderate skew-surge climatology, prevents successive extreme storm-tide events from happening within 4–10 d of each other. Of 85 extreme storm-tide events, only once did two extreme storm tides occur less than 4 d apart due to two closely spaced storms straddling a peak spring tide period. Generally, there are at least 10 d between extreme storm-tide events.

Extreme events caused by “blocking” weather types coincided with storm centres located in the north of NZ and predominantly impacted the northeast coast and occasionally the west coast of the North Island. Extreme events caused by “trough” weather types coincided with storm centres that track further south, across or south of NZ and impacted mainly the South Island and the west coast of the North Island.

Lower numbers of extreme storm-tide and skew-surge events were observed in late spring to early summer (which is also outside the ex-tropical cyclone season). This reflects a tendency for more extreme surges earlier in the year but was also noticeably influenced by the mean annual sea-level cycle that peaks in the austral autumn in most places. There is no apparent relationship between extreme storm-tide events and large-scale climate-variability modes such as ENSO. The considerable influence of the relatively low-amplitude mean sea-level anomaly shows that a relatively small SLR will drive rapid increases in the frequency of presently rare extreme storm tides.