the Creative Commons Attribution 4.0 License.
the Creative Commons Attribution 4.0 License.
Spatial assessment of probable recharge areas – investigating the hydrogeological controls of an active deep-seated gravitational slope deformation
Thomas Zieher
Jan Schmieder
Thom Bogaard
Martin Rutzinger
Christoph Spötl
Continuous and slow-moving deep-seated landslides entail challenges for the effective planning of mitigation strategies aiming at the reduction of landslide movements. Given that the activity of most of these landslides is governed by pore pressure variations within the shear zone, profound knowledge about their hydrogeological control is required. In this context, the present study presents a new approach for the spatial assessment of probable recharge areas to better understand a slope's hydrogeological system. The highly automated geo-statistical approach derives recharge probability maps of groundwater based on stable isotope monitoring and a digital elevation model (DEM). By monitoring stable isotopes in both groundwater and precipitation, mean elevations of recharge areas can be determined and further constrained in space with the help of the DEM. The approach was applied to the Vögelsberg landslide, an active slab of a deep-seated gravitational slope deformation (DSGSD) in the Watten valley (Tyrol, Austria). Resulting recharge probability maps indicate that shallow groundwater emerging at springs on the landslide recharges between 1000 and 1650 m a.s.l. In contrast, groundwater encountered in wells up to 49 m below the landslide's surface indicates a mean recharge elevation of up to 2200 m a.s.l. matching the highest parts of the catchment. Further inferred proxies, including flow path length, estimated recharge area sizes, and mean transit times of groundwater, resulted in a profound understanding of the hydrogeological driver of the landslide. It is shown that the new approach can provide valuable insights into the spatial pattern of probable recharge areas where mitigation measures aiming at reducing groundwater recharge could be most effective.
- Article
(13362 KB) - Full-text XML
- BibTeX
- EndNote
The variability in slow but continuous movements of large-scale, deep-seated gravitational slope deformations (DSGSDs) poses a serious risk to anthropogenic infrastructure and therefore threaten human needs in mountain areas (Crosta et al., 2013; Cignetti et al., 2020). In many cases, secondary subunits show enhanced activity forced by pore pressure changes related to groundwater variations (Lacroix et al., 2020). Mitigation measures aiming at the reduction of the pore pressure provide a promising tool to decrease the activity of moving slopes (Eberhardt et al., 2007; Hofmann and Sausgruber, 2017). In this context, a detailed understanding of the hydrogeological processes controlling pore pressure variations is an essential requirement to enhance the effectiveness of mitigation strategies. It is important to know where the landslide-controlling groundwater originates and which subsurface flow path(s) it takes until the water reaches the landslide's governing aquifer or until the pore pressure wave within the saturated zone reaches the landslide (Bogaard and Greco, 2016). Only then is it possible to develop solutions to effectively drain a slope in order to reduce a landslide's activity. The performance of nature-based solutions may strongly be enhanced by a complete understanding of a landslide's driver (Kumar et al., 2021b, a).
Groundwater recharge is controlled by precipitation and/or surface water percolating through the unsaturated zone towards the aquifer. Flow within the aquifer is further controlled by gravity and its hydraulic properties (Welch and Allen, 2014). Various methods can be used to characterize groundwater flow dynamics, storage, and recharge areas including (i) hydrochemistry (Cervi et al., 2012), (ii) hydromechanical modelling (Cappa et al., 2004), (iii) reactive and conservative tracer tests (Bogaard et al., 2007; Vallet et al., 2015; Hilberg, 2016; Ronchetti et al., 2020), and (iv) non-invasive geophysical methods (Jomard et al., 2007; Siemon et al., 2009; Chalikakis et al., 2011; Zieher et al., 2017; Lajaunie et al., 2019). The use of tracers to track water through the slope's subsurface entails an auspicious way to assess and characterize the hydrogeological forcing of a landslide. Natural and artificial tracers have been widely used to obtain insights into the hydrogeological setting of deep-seated slides (Montety et al., 2007; Vallet et al., 2015; Strauhal et al., 2016; Koltai et al., 2018). Whereas the use of artificial tracers relies on a well-designed experimental set-up and assumptions of potential flow mechanisms, the advantage of natural tracers is that their availability is ubiquitous and tied to natural processes of groundwater recharge (e.g. rainfall and snowmelt) (Binet et al., 2007; Ronchetti et al., 2020).
The stable isotopic composition of water is a widely used conservative tracer applied to a wide range of hydrological studies at different scales and with different objectives. On a global scale, Jasechko et al. (2014) investigated stable isotope ratios in precipitation and groundwater to determine the seasonal differences in groundwater recharge. On a regional scale, Blasch and Bryson (2007) identified seasonality and the dominating areas of recharge within several subbasins by using stable isotope values of precipitation and groundwater. On a catchment scale, Schmieder et al. (2016) exploited stable isotopic signatures combined with a physically based snow model for hydrograph separation and quantification of snowmelt contributions to streamflow. Subsequently, for better understanding and quantification of landslide hydrology, a stable-isotope-supported characterization of recharge, constraining groundwater flow systems, and estimating mean recharge elevations are proven methodologies (Scanlon et al., 2002; Bouchaou et al., 2008; Guglielmi et al., 2002; Mikoš et al., 2004). The underlying principle is the altitude-dependent fractionation of stable hydrogen and oxygen isotopes in precipitation creating a systematic decrease in the abundance of 18O with increasing elevation (Dansgaard, 1964). Comparing the stable isotopic composition of groundwater sampled at springs or in wells with that in precipitation allows the estimation of mean recharge elevations (Moser and Rauert, 1980; Blasch and Bryson, 2007). Many studies utilized this “altitude effect” to infer flow paths between identified recharge elevation and discharge location (e.g. a spring) along a simple 2D transect (Guglielmi et al., 2002; Madritsch and Millen, 2007; Hilberg and Riepler, 2016). Commonly, the result of such studies is a conceptualized hydrogeological model describing the groundwater flow along the slope.
Guglielmi et al. (2002), for example, investigated the groundwater recharge mechanisms of two different deep-seated landslides in the French Alps. For both landslides, they differentiated a shallow and a deep-seated groundwater flow. A hydrogeological study presented by Madritsch and Millen (2007) evidenced groundwater flow paths along a continuous basal shear zone ranging from the highest elevations within a DSGSD towards its toe. Furthermore, the authors affirmed the importance of having a local δ18O-precipitation altitude gradient for the assessment of recharge areas. This is supported by results presented by Liebminger et al. (2006), who determined distinct differences in the isotopic composition of precipitation on the margin of the Alps compared to inner-alpine settings. Cervi et al. (2012) identified the origin of groundwater and assessed deep groundwater inflow into a landslide using a wide range of methods including hydrochemistry and in situ monitoring. Cappa et al. (2004) coupled hydromechanical modelling with long-term hydrochemical monitoring at a large moving rock slope to investigate the influence of location and amount of water infiltration on the landslide's hydromechanical behaviour. The authors of these studies described the hydrogeological mechanisms forcing deep-seated landslides. However, studies employing the monitoring of stable isotopes typically lack locally validated δ18O altitude gradients based on periodical sampling of precipitation across the respective elevation range (Madritsch and Millen, 2007; Liebminger et al., 2006). Another knowledge gap identified in the literature concerns the area-wide and quantitative analysis of probable recharge areas (Vallet et al., 2015; Ronchetti et al., 2020). The present study addresses these gaps by combining local isotopic precipitation, groundwater time series, and coherent geodata to assess areas of groundwater recharge throughout the landslide's catchment area. Resulting maps of probable recharge areas are seen as an opportunity to enhance the understanding of the hydrogeologic drivers of deep-seated landslides required for planning effective drainage measures.
In this study we introduce and apply a novel geo-statistical approach for the 3D delineation of probable groundwater recharge areas. The approach is applied to the deep-seated Vögelsberg landslide in the Watten valley (Tyrol, Austria). This paper is going to (a) present the geo-statistical approach and the required hydrogeological monitoring of groundwater and precipitation, (b) derive the local altitude gradient of δ18O in precipitation and compare it against regional datasets, (c) identify probable recharge areas of the landslide-controlling groundwater, and (d) establish a conceptual model of the hydrogeological controls of the landslide.
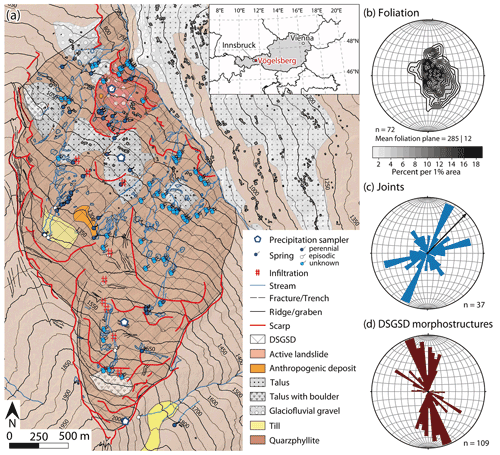
Figure 1Geological map of the study area (a), stereographic contour plot of foliation with pole points (b), and rose plots indicating the orientation of joints (c) and DSGSD morphostructures including scarps, fractures, trenches, ridges, and grabens (d) (DTM and spring inventory: Federal State of Tyrol, geological basemap adapted from GBA).
The NE-facing slope at the entrance of the Watten valley in Tyrol (European Alps, Austria) is affected by a deep-seated gravitational slope deformation (DSGSD) with an approximate N–S extent of 3 km and E–W extent of 1.6 km (Fig. 1a). This DSGSD ranges from 750 to 2150 m a.s.l., wherein an active part (ca. 500×500 m) is situated on the foot-slope between 750 and 1050 m a.s.l. A N–S striking ridge represents the western and upper boundary of the topographic DSGSD catchment. The ridge rises from 1100 m in the north to 2200 m in the south. The Wattenbach draining the Watten valley limits the DSGSD towards the east at an elevation between 750 m and 950 m a.s.l. in the study area. The latter belongs to the Austroalpine Innsbruck quartz phyllite complex consisting of early Paleozoic greenschist-grade meta-sediments dominated by quartz phyllite (Rockenschaub et al., 2003).
2.1 Hydrogeological characterization
Outcrops within the study area are restricted to quartz phyllites in which intercalations of marble layers varying in thickness from a few centimetres to several metres are common. The orientation of the foliation is uniform across the slope and slightly dipping towards WNW (Fig. 1b). Joints are abundant, showing a preferred orientation of open and sub-vertical joints along NNE–SSW and ENE–WSW (Fig. 1c). Extensional morphological features such as double-crested ridges, grabens, trenches, open fractures, and scarps of different generations are present along and in proximity to the ridge, marking the upper western boundary of the DSGSD. The orientation of most of these morphostructures is in agreement with the NNW–SSE striking ridge (Fig. 1d). Heavily fractured bedrock dominates the ridge. Till and fluvial gravel are present in local morphological depressions on the DSGSD.
The fractured quartz phyllite constitutes the main aquifer providing flow paths. Due to the high density of well-connected fractures this aquifer is assumed to provide isotropic flow similar to porous aquifers (Guglielmi et al., 2002). Thin and patchy Quaternary sediments might act as small local porous aquifers or aquitards depending on their grain size. Shear zones and landslide slip surfaces are assumed to induce a strong directional hydraulic heterogeneity along which the hydrogeological properties deviate strongly from those of the surroundings. While shear zones in quartz phyllite create low-permeability zones, brittle deformation commonly increases permeability along densely fractured shear zones (Binet et al., 2007; Bonzanigo et al., 2007).
The highest springs draining the DSGSD can be found 150 to 350 m below the respective ridge elevation. This is the case at 1200 m a.s.l. for the northernmost part and at 1700 m a.s.l. for the southernmost part of the DSGSD. In some cases, the spring water follows the stream network only for a short distance and infiltrates into the subsurface further downslope. This phenomenon can especially be observed during snowmelt and at the uppermost springs (approx. >1500 m a.s.l.). They tend to fall dry after long periods without precipitation or snowmelt. On the other hand, springs at lower elevations (<1350 m a.s.l.) show continuous discharge. In general, this lower area is characterized by a high density of springs resulting in a dense network of small creeks. The mean perpendicular distance between the five creeks draining the DSGSD is 250 m, while the mean spring density is one spring per 130×130 m.
2.2 Hydro-meteorological landslide forcing
In a previous study Pfeiffer et al. (2021) showed that phases of landslide acceleration occur as a delayed response to long-lasting rainfall and/or snowmelt events. Constant displacement rates of 1–2 cm yr−1 prevail most of the year, while non-seasonal and extraordinary hydro-meteorological events result in mean rates of up to almost 6 cm yr−1. Results of a hydro-climatological model indicate an annual average of 644 mm of water (snowmelt + rainfall − evapotranspiration) in the catchment area of the landslide between 2009 and 2018. Hydro-meteorological events of up to 600 mm of water within 100–200 d triggered phases of accelerated landslide movement. Pfeiffer et al. (2021) also show that the landslide's response time varies depending on the type of water source. While spatially heterogeneous snowmelt induces a relatively fast (0–8 d) response, spatially evenly distributed rainfall entails a delayed (approximately 45 d) response of landslide acceleration. Computed spatially distributed response times suggest a spatially varying delay in the case of acceleration phases triggered by snowmelt. For example, in early 2019 snowmelt on and right above the landslide occurred only a few days before the respective landslide acceleration. In contrast, in areas above 1700 m snowmelt occurred after the acceleration phase and therefore likely did not contribute to the landslide's short-term dynamics (Pfeiffer et al., 2021).
3.1 Field work
Detailed hydrogeological mapping by the Landesgeologie department of the Federal State of Tyrol in 2016 provided valuable information about the location of creeks and springs. Additional field mapping was carried out in 2019 by paying special attention to geological and morphological structures bearing essential information on the subsurface hydrodynamic behaviour. The orientation of foliation and joint surfaces observed at bedrock outcrops was recorded using a geological compass. Morphological structures associated with DSGSD activity (e.g. scarps, counter scarps, extensional fractures, and trenches) were mapped in the field and digitized with the help of a shaded relief image derived from a digital terrain model (DTM) based on airborne laser scanning (ALS) by the Federal State of Tyrol.
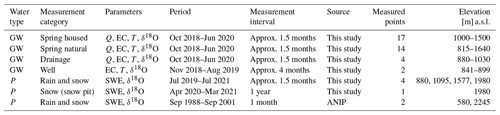
Precipitation was collected between July 2019 and July 2021 using four Palmex (http://www.rainsampler.com, last access: 7 June 2022) samplers (Gröning et al., 2012) mounted on a pole and installed at 880 m, 1095 m, 1577 m, and 1980 m a.s.l. (Fig. 2a and c). Water samples were collected during each field campaign, and the amount of rain and melted snow was recorded. At the end of the winter season (15 April 2020 and 30 March 2021), snow pits were dug at the uppermost location (1980 m a.s.l.) to estimate the snow water equivalent (SWE) by weighting snow samples within a known volume. Snow samples were collected for isotopic analysis.
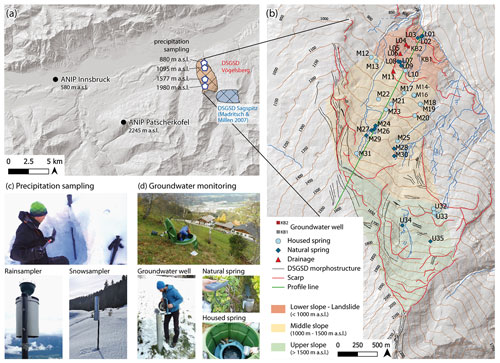
Figure 2Overview of the hydrogeological monitoring. (a) Study area and nearby Austrian Network for Isotopes in Precipitation (ANIP) stations at Innsbruck and Patscherkofel; (b) map of monitoring sites and locations of measurement points selected for monitoring; (c, d) fieldwork photographs of precipitation and groundwater sampling (DTM source: Federal State of Tyrol).
As an independent reference, monthly precipitation data from the Austrian Network for Isotopes in Precipitation (ANIP) stations at Innsbruck (580 m a.s.l. and 18 km from the study area) and Patscherkofel (2245 m a.s.l. and 13 km from the study area) were used, providing a complete time series from 1 September 1988 to 1 September 2001 (Fig. 2a). Hydrogeological monitoring campaigns were carried out from October 2018 until June 2020. Based on the hydrogeological inventory provided by the Federal State of Tyrol (Fig. 1a), 35 measurement points fulfil the demands to be part of a temporally condensed measurement set-up. Selection of measurement points was done based on the following criteria: measurement points are accessible and permitted to be accessed by the owner during the monitoring period, natural water outlets are effectively measurable without disturbance of the surrounding environment, and measurement points intersect the assumed area of potential landslide influence. The assumed area of potential landslide influence covers the lowest and highest part of the DSGSD and is grouped into three elevation bands (Fig. 2b). Measurement point designation is based on the discharge elevation, and the prefixed acronym indicates the elevation band. Measurement points L01–L10 intersect with the sections of the lower slope and the area of the active landslide (<1000 m a.s.l.). Measurement points M11–M31 intersect with the middle slope section (1000–1500 m a.s.l.) and U32–U35 with the upper slope (>1500 m a.s.l.).
Multi-temporal measurements of discharge (Q in L s−1), water temperature (T in ∘C), and electrical conductivity (EC in µS cm−1) were conducted at 35 measurement points including natural springs (n=14), housed springs (n=17), and drainages (n=4) within the DSGSD (Fig. 2b). Housed springs are structurally supported groundwater outlets and relevant for residents' water supply. Natural springs are mostly diffuse zones of groundwater exfiltration. Discharge was measured using a bucket and a stop watch. EC and T were measured in the field using a WTW Cond 3310 device (Fig. 2d). Water samples for stable isotope analyses were periodically taken from selected springs and drainages. Furthermore, T and EC measurements, as well as multiple water samples, were obtained from two groundwater wells (KB1 and KB2) located on the active part of the landslide. The measurements and samples were taken at various depths using a sampling probe on 29 November 2018, 16 April 2019, and 13 August 2019. Screens allowing groundwater to enter the wells are installed at 16 to 49 m depth in KB1 and at 21 to 39 m depth in KB2. Well measurements and sampling were done at constant intervals from the piezometric height towards the bottom of the well. Both wells are equipped with piezometers recording the groundwater level and are operated by the Austrian Service for Torrent and Avalanche Control. Table 1 summarizes acquired and utilized field data and their measurement properties.
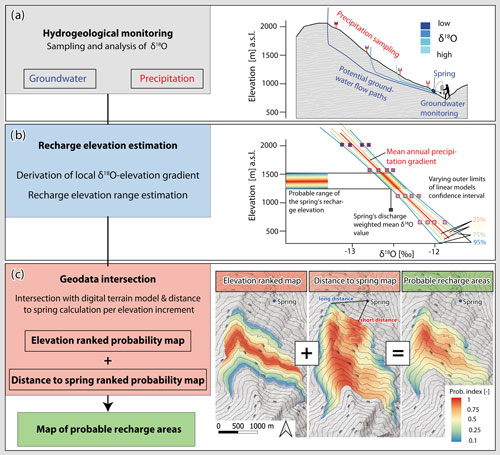
Figure 3Proposed workflow for deriving maps of probable recharge areas including (a) hydrogeological monitoring, (b) recharge elevation estimation, and (c) geodata intersection (DTM source: Federal State of Tyrol).
Precipitation samples of snow, rain, and groundwater were analysed regarding their oxygen isotopic composition. Samples were stored at 4 ∘C before being analysed with a Picarro L2140-i cavity ring-down spectroscopy analyser following the procedures outlined by van Geldern and Barth (2012). Results are reported in per mill (‰) against the Vienna Standard Mean Ocean Water (VSMOW). Calibration of the instrument was accomplished using VSMOW2, GISP2, and SLAP standards. The long-term analytical precision of measurements is 0.1 ‰ (1σ). Acquired hydrogeological monitoring data serve as the basis for applying the subsequently presented approach for assessing probable recharge areas (Fig. 3a).
3.2 Estimation of inverse transit time proxy
The inverse transit time proxy (ITTP) proposed by Tetzlaff et al. (2009) was used to estimate groundwater transit times. The ITTP is defined as the ratio of the standard deviation of δ18O in groundwater to the standard deviation of δ18O in precipitation. The proxy reflects the degree of attenuation of annually varying δ18O of precipitation in groundwater for which a lower ratio indicates a higher attenuation and a longer transit time. The ITTP was calculated for every groundwater sample that had at least five multi-temporal δ18O measurements available for calculating the standard deviation. Standard deviations from multi-temporal δ18O ratios in precipitation were calculated from ANIP and Vögelsberg samples.
3.3 Estimation of probable recharge elevations
Mean recharge elevations were estimated for each spring and groundwater sample using the altitude effect of oxygen isotopes in precipitation (Moser and Rauert, 1980; Clark and Fritz, 1997; Mook, 2006). This effect has been applied in several landslide case studies and requires a precise estimation of the local δ18O altitude gradient of precipitation which can be described by the linear model's coefficients β (slope) and α (intercept) (Madritsch and Millen, 2007; Vallet et al., 2015; Hilberg and Riepler, 2016). Two local annual δ18O altitude gradients of precipitation were derived for the time spans July 2019 to June 2020 and June 2020 to July 2021 (referred to as and Vögelsberg gradients). Furthermore, 13 annual altitude gradients were computed from the ANIP δ18O time series covering the period from September 1988 to September 2001. The ANIP altitude gradients show good agreement with the locally derived and Vögelsberg gradients (Sect. 4.3). Therefore, all δ18O time series were used to establish a linear model of the δ18O ratio against elevation, considering weights according to the precipitation amount. Furthermore, the probability distribution and respective 95 % confidence bands were computed as lower and upper boundaries of this long-term gradient, representing the inter-annual variability in δ18O in precipitation.
For subsequently estimating the mean recharge elevation (MRE) of a groundwater sample, the respective isotope ratio δ18OGW was passed to the linear equation of the long-term δ18O altitude gradient of precipitation:
where α and β are the coefficients of the derived long-term gradient. By considering the gradient's level of confidence a probability distribution of mean recharge elevations was deduced for each groundwater sample (Fig. 3b). This probability distribution was then scaled to a range of 0 to 1 in which 0 represents a low and 1 a high recharge elevation probability.
3.4 Geodata-supported approach for the derivation of probable recharge areas
The recharge elevation provides essential information about a slope's governing hydrogeological system (Guglielmi et al., 2002). Furthermore, the probability distribution of recharge elevation enhances the potential for further analysis towards a spatial assessment of probable recharge areas. In this context we present a new method to constrain probable recharge areas by transferring the stable-isotope-based recharge elevations into the third dimension. This requires a digital terrain model (DTM) representing the ground surface elevation of each raster cell within a catchment, the xyz coordinates of groundwater sampling points, and the respective probability distributions of recharge elevations. These elevation distributions (Sect. 3.3) were first compared against the elevation information of the DTM. An elevation-ranked recharge probability map was compiled by transferring the probability values to the respective recharge elevations (Fig. 3c). Raster cells with the value of 1 represent the highest probability and raster cells with the value of 0 the lowest probability.
In order to discriminate between potential recharge areas at the same elevation but differing in proximity to the discharge location, a second distance-dependent indicator was introduced. Based on the assumption that water flow along the shorter and direct path between recharge and exfiltration is more likely, 3D distances were stepwise calculated between every raster cell and the discharge location. 3D distances of the same elevation step were ranked by scaling the distances to a spring to a range of 0 to 1, the distance-to-spring index. A value of 0 represents the maximum distance and a value of 1 the minimum distance to the respective spring per elevation band. Introducing a maximum limit defined by a multiplier of the minimum distance to the spring per elevation band yields a fan-shaped area of flow path distance-dependent recharge probability.
The combination of the elevation-ranked recharge probability map and the map showing the distance to spring index results in a map of probable recharge areas. This is accomplished by adding the two maps and applying a 0–1 scaling. The resulting recharge probability maps demonstrate the unique possibility to further infer potential groundwater flow path lengths specified as the 3D distance between the maximum value of the recharge probability map and its discharge location. Aggregation of individual recharge probability maps using mean values creates a single recharge probability map covering recharge information of all investigated groundwater.
We further used discharge measurements (Q) for each spring to estimate the size of the potential recharge area (A) provided that hydroclimatological input data (I) are available. In the case only a fraction of the water infiltrates into the subsurface, an additional coefficient Ic is introduced. Ic varies between 0 and 1, denoting 0 % and 100 % infiltration, respectively (Cronshey et al., 1986):
The estimated size of the recharge area was transferred to the recharge probability map indicating the concordant area with the highest probability values. The described workflow for the assessment of probable recharge areas was automated using the R programming language (R Core Team, 2021). A DTM in the same coordinate reference system as the location of the groundwater discharge locations and at a 5 m spatial resolution was used within the workflow.
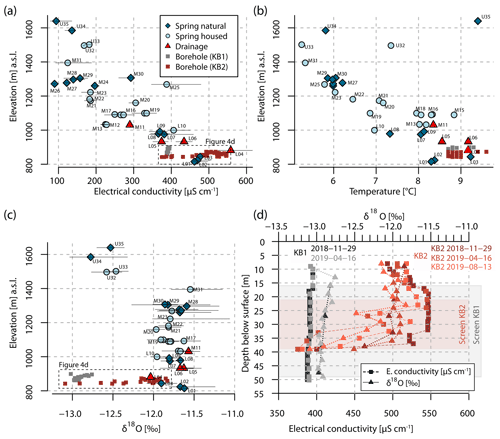
Figure 4Diagrams showing mean values of EC (a), T (b), and δ18O (c) of monitored springs and boreholes. Thin black lines in (a) and (c) indicate the observed range between minimum and maximum values. EC and δ18O measured at different depths in the KB1 and KB2 wells are shown in (d). The groundwater level during sampling can be obtained from Fig. 6.
4.1 Electrical conductivity and water temperature
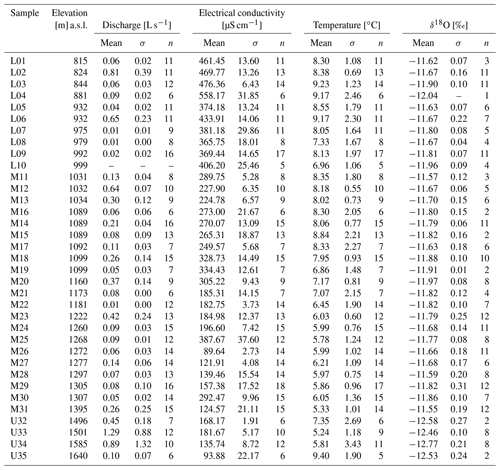
The EC of selected springs and drainages shows variations in space and time. A spatial variation between 80 and 600 µS cm−1 of absolute EC values was observed at different measurement points. Lower values in the order of 80–150 µS cm−1 are commonly observed at the uppermost springs, while samples from lower elevations show values between 400 and 600 µS cm−1 (Fig. 4a, Table 2). Measurements of EC along the KB1 well show values up to 395 µS cm−1 for the upper part and 388 µS cm−1 for the lower part (49 m below the surface). The KB2 well shows larger variations with 550 µS cm−1 close to the surface and 375 µS cm−1 at 39 m depth (Fig. 4d). The high values of 550 µS cm−1 are in agreement with data from nearby drainages.
The annual range of EC at individual measurement points is in the range from 5 to 132 µS cm−1. For the majority of measurement points (31 out of 35) the temporal variability is below 66 µS cm−1. In general, minimum values were observed in spring and early summer, whereas maximum values occur in autumn and winter. No distinct temporal variability in EC was observed within the KB1 well (variability < 2 µS cm−1). EC values between the measurement campaigns in KB2, on the other hand, vary up to 25 µS cm−1 at comparable depths.
The mean T of groundwater ranges between 8 and 10 ∘C at lower sites and 5 and 6 ∘C at higher locations (Fig. 4b, Table 2). A constant T of 8.7 ∘C was measured within the lowest 39 m of the KB1 well. A tendency towards higher T values of up to 9 ∘C is observable within the uppermost part of this well. Temperatures in the KB2 well show similar values between 8.7 and 9 ∘C. The T of the wells is in general agreement with that of nearby springs.
The water T of most of the springs follows a seasonal pattern with higher values during summer and lower values during winter. The difference between annual minima and maxima reaches up to 5 ∘C. The water T within the wells is constant over time with variation below 0.2 ∘C in KB2 and no measurable differences in KB1.
4.2 Discharge, inferred recharge area sizes, piezometric height, and landslide movement
The annual mean discharge of all measurement points is on average 0.2 L s−1. The majority of them (34 out of 35) show mean values of below 1.0 L s−1 (Table 2). Respective annual means indicate recharge area sizes ranging from about 300 to 250 000 m2 for the individual discharge locations and variable Ic (Eq. 2) (Fig. 5).
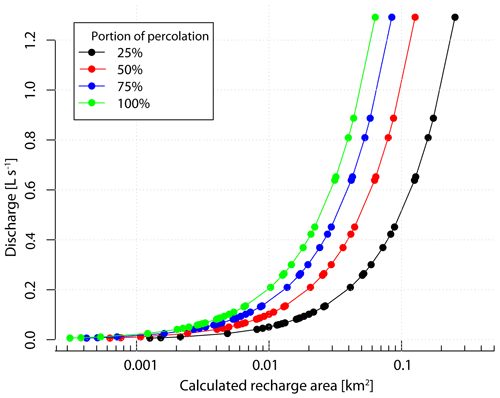
Figure 5Estimated size of recharge area based on average discharge and annual water input of 643 mm (Pfeiffer et al., 2021). The recharge area size was derived from Eq. (2) for four different infiltration ratios Ic (25 %, 50 %, 75 %, and 100 %).
Generally low discharge was observed in autumn 2018 and in summer 2019. Comparably high discharge was measured in spring and early summer 2019 and coincided with a period of intense snowmelt which led to landslide accelerations (Pfeiffer et al., 2021). Within this period, maximum discharge at springs located at lower elevations (e.g. spring M14 at 1090 m a.s.l.) occur up to almost 3 months earlier than the maximum discharge of springs located at higher elevations (e.g. spring M31 at 1395 m a.s.l.). This phenomenon is evidenced by comparing time series of scaled spring discharge values (Fig. 6). The temporal variability in discharge at springs at lower elevations and closer to the active discharge is in conformity with time series of piezometric heights. Piezometric heights measured in KB1 show a temporal variability of approximately 1 m between 2018 and 2020 and conformity with a time series of mean displacement rates (Fig. 6). Phases of accelerated landslide movements therefore coincide with a higher piezometric level and higher discharge at springs close to the landslide. The accelerated landslide movements during the period of higher water levels and increased spring discharge in early 2019 were the response to intensive snowmelt as shown by a physically based snow model (Pfeiffer et al., 2021). The aquifer response to subsequent snowmelt and summer precipitation events is indicated by the comparison of respective groundwater time series with precipitation time series at the close-by Patscherkofel station (Fig. 6).
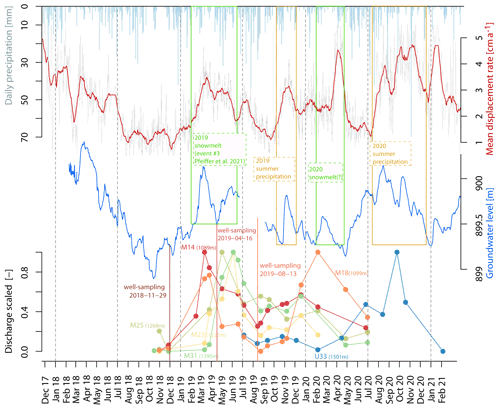
Figure 6Time series of daily precipitation at Patscherkofel (source: ZAMG), mean displacement rate (source: Federal State of Tyrol), groundwater level in well KB1 (source: Austrian Service for Torrent and Avalanche Control), and discharge of selected housed springs. Discharge was scaled between 0 and 1 for better comparability of the different springs.
4.3 δ18O in precipitation
Maximum inter-annual variations in the stable isotopic composition of precipitation were observed with δ18O ratios between −3 ‰ and −20 ‰ at the uppermost precipitation sampling location (Fig. 7b). Furthermore, the precipitation samples show a distinct seasonal pattern throughout the year with maximum values of δ18O occurring in summer and autumn and minimum values in winter and spring.
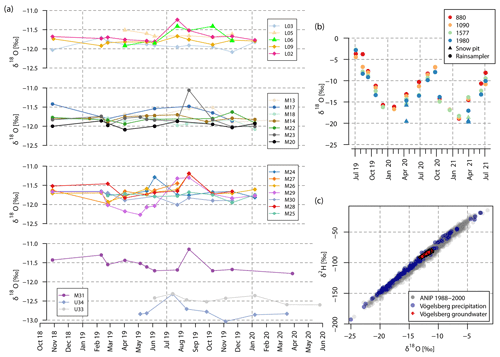
Figure 7Time series of δ18O of selected springs (a), time series of δ18O in precipitation (b), and δ18O vs. δ2H in precipitation (ANIP and Vögelsberg) and groundwater (Vögelsberg) (c).
Analyses of the stable isotopic composition of precipitation sampled at different elevations within the study area indicate a clear decrease in δ18O with increasing elevation. Whereas the annual δ18O ratios weighted by the respective amount of water sampled at the uppermost (1980 m) station are between −13.19 ‰ and −13.71 ‰, values at the lowermost station (880 m a.s.l.) are between −11.29 ‰ and −11.31 ‰ (Fig. 8a). Time series of annually weighted δ18O in precipitation recorded at ANIP's Patscherkofel station (2245 m) range between −12.28 ‰ and −14.18 ‰ and at the Innsbruck station (580 m) between −10.11 ‰ and −12.16 ‰ within the 13-year record. Linear regressions of all on-site sampled data points result in altitude gradients of 0.21 ‰ per 100 m () and 0.16 ‰ per 100 m (). Values of on-site δ18O were extrapolated, utilizing derived gradients. Resulting values indicate conformity of the on-site data with respective ANIP reference data (Fig. 8b).
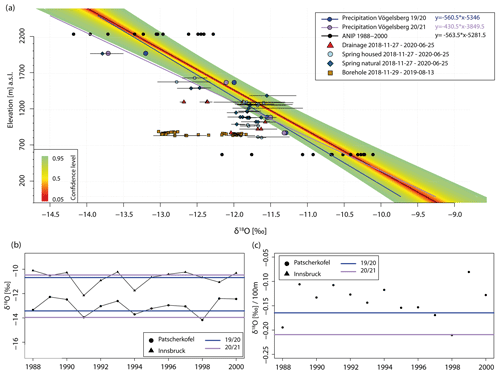
Figure 8δ18O values and elevation of precipitation and groundwater samples. (a) Precipitation gradients, confidence interval, and groundwater samples. (b) Time series of amount-weighted annual δ18O in precipitation at ANIP stations Patscherkofel and Innsbruck (data retrieved from https://wasser.umweltbundesamt.at/h2odb/, last access: 20 December 2021). Annual δ18O values (extrapolated to the corresponding ANIP station elevation) of the study are shown by horizontal lines. (c) Time series of annual δ18O-precipitation gradients.
A total of 13 annual gradients were derived from the ANIP time series showing a range between 0.21 ‰ per 100 m and 0.08 ‰ per 100 m (Fig. 8c). The 95 % confidence interval (Fig. 8a) of ANIP δ18O ratios indicates a range of −13.30 ‰ to −12.57 ‰ for the uppermost on-site sampling station at 1980 m a.s.l. and −11.25 ‰ to −10.54 ‰ for the lowermost on-site station at 880 m a.s.l. The calculated mean annual δ18O values for the Vögelsberg sampling stations at 880 m, 1095 m, and 1577 m a.s.l. are within the determined range of the 95 % confidence limit from the ANIP reference gradients. Only the δ18O value for the uppermost sampling location (1980 m a.s.l.) from is below the 95 % confidence limit. Consequently, the slope of the fitted linear model of −0.21 ‰ per 100 m representing the altitude gradient for the same period is exceptionally high compared to the long-term average of −0.14 ‰ per 100 m (Fig. 8c). The slope of the Vögelsberg gradient for the sampling period (−0.16 ‰ per 100 m), however, is very close to the long-term mean (−0.14 ‰ per 100 m).
4.4 δ18O in groundwater
The isotopic composition of groundwater samples was analysed for the period from November 2018 to June 2020. Within this period the mean δ18O ratios of drainages and springs below 1450 m a.s.l. range from −12.0 to −11.5 ‰ (Table 2). No distinct differences in the isotopic composition of the water within the wells (KB1 and KB2) were observed between the measurement campaigns. Springs located above 1450 m a.s.l. show lower mean values of about −12.5 ‰ (Fig. 4c). The lowest values (−13 ‰) were measured in the KB1 well. Samples from this well show more constant δ18O ratios along the vertical profile compared to KB2. KB2 on the other hand shows a similar isotopic composition as the surrounding springs (approx. −12 ‰) within the upper part of the well and converges towards lower δ18O ratios (−13 ‰) deeper down (Fig. 4d).
The temporal variability expressed as the difference between δ18O maxima and minima is less than 1 ‰ for all water samples (Fig. 7a). Springs discharging at high and middle elevations (1200–1700 m a.s.l.) show a higher temporal variability (average: 0.6 ‰) than springs located at lower parts of the slope and the active landslide area (average: 0.2 ‰).
Considering both δ18O and δ2H values, the groundwater samples are aligned along the precipitation data and therefore agree with the local meteoric water line. All groundwater samples are evenly surrounded by higher and lower δ18O and δ2H precipitation values indicating a balanced seasonal recharge (Fig. 7c).
4.5 Inverse transit time proxy
The seasonal amplitude of δ18O in precipitation in Alpine regions shows differences of up to 20 ‰ between monthly maxima and minima (Liebminger et al., 2006). Time series of δ18O in groundwater within the study area on the other hand indicate a strong attenuation of this input signal in the subsurface. The level of attenuation is reflected by the ITTP after Tetzlaff et al. (2009). Calculated ITTPs for all groundwater samples with more than 5 measurements show standard deviation ratios below 0.1. According to Tetzlaff et al. (2009), who compared ITTP values and published mean transit times (e.g. McGuire et al., 2005; Rodgers et al., 2005a, b; Tetzlaff et al., 2007), a ratio below 0.1 indicates transit times of at least half a year. Groundwater samples in the study area reach ITTP values indicating transit times of up to 3.5 years.
4.6 Recharge probability map
δ18O ratios from springs below 1450 m a.s.l. and in the active landslide (750 to 1050 m a.s.l.) indicate mean recharge elevations between 1000 m and 1650 m a.s.l. (Fig. 8a). Samples from the wells with values as low as −13 ‰, on the other hand, indicate mean recharge elevations of up to 2200 m a.s.l. representing the highest and southernmost parts of the DSGSD. Differentiation of individual recharge areas was accomplished using the geodata-supported approach (Sect. 3.4). Spatial patterns of recharge areas become apparent by comparing recharge probability maps obtained for each discharge location. Differences can be observed by comparing recharge maps of two springs located at approximately 840 m and 820 m a.s.l. at a distance of 45 m. While one spring (L03) has its recharge area in the highly fractured more northern part of the ridge at an average elevation of 1350 m a.s.l., the other springs (L01 and L02) receive water recharging closer to the landslide at 1170 m a.s.l. (Fig. 9a and b).
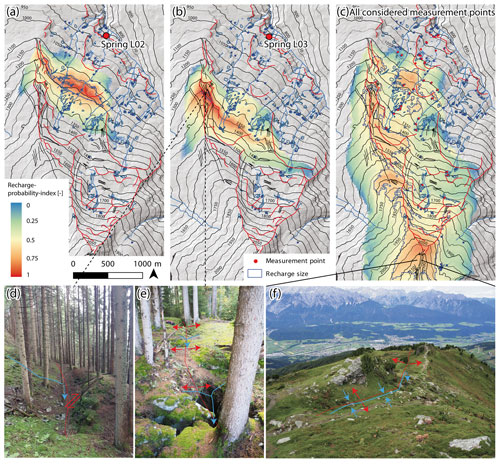
Figure 9Maps of probable recharge. Panels (a) and (b) show the recharge probability index for selected springs draining the active landslide, and (c) shows an aggregated map considering recharge probabilities for all spring, drainage, and well samples. Panel (d) shows a picture of an upslope-facing scarp assumed to represent a recharge zone (assumed flow paths indicated by blue arrow lines), as well as (e) open tensional fractures and (f) a dry valley between a double-crested ridge (DTM source: Federal State of Tyrol).
The aggregated map including recharge areas of all discharge locations (Fig. 9c) shows that within the probable recharge elevation range from 1200 m up to 2200 m a.s.l. recharge is biased towards convex-shaped landforms as opposed to concave-shaped landforms. The former are commonly characterized by a dense network of fractures. The prominent fractured ridge marking the western boundary of the DSGSD (Fig. 9d–f) therefore seems to act as a preferential recharge zone, while groundwater exfiltration occurs preferentially in concave-shaped landforms.
4.7 Hydrogeological landslide control
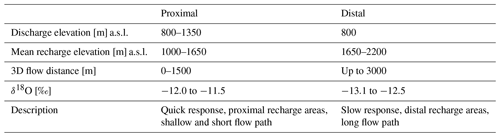
Based on the maps of probable recharge areas it is evident that groundwater recharges over a large extent of the landslide's upslope catchment area. Whereas springs located on the landslide (e.g. L02 and L03) infer medium 3D flow distances in the order of 800 to 1400 m, those inferred for the well samples reach up to 3000 m (Fig. 10). Consequently, pore pressure changes, triggering landslide activity, are controlled by a distal and a proximal forcing of groundwater recharge. Comparing inferred 3D flow distances of all springs against their discharge elevation, it becomes evident that the inferred flow distance increases with decreasing elevation (Fig. 10). Moreover, springs characterized by longer flow distances indicate longer transit times reflected by smaller ITTP compared to springs with a close-by recharge area and higher ITTP. Simultaneously, this observation is reminiscent of a similar pattern between increasing EC and decreasing discharge elevation (Fig. 4a).
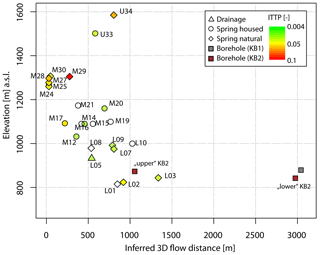
Figure 10Relation between inferred 3D flow distance and discharge elevation. Colours represent calculated ITTP following Tetzlaff et al. (2009) for which calculations were feasible. Sampling dates are in conformity with data points in Fig. 7a.
These observations suggest the existence of a homogeneous flow in a fractured aquifer of rather uniform hydrodynamical properties (Fig. 11). Recharge of this aquifer takes place between 1000 and 1650 m a.s.l. (Fig. 9c). The upper boundary of this groundwater flow system coincides with elevations representing the ridge associated with the landslide, suggesting a topographically controlled and almost slope-parallel flow system.
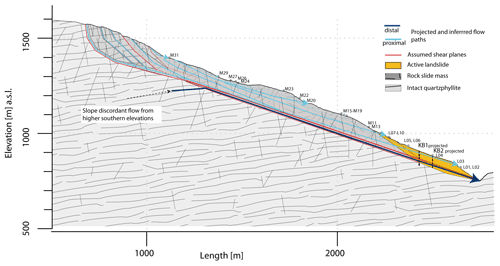
Figure 11Conceptual hydrogeological model summarizing dominant groundwater flow systems along a transect through the DSGSD and Vögelsberg landslide.
Another water transport mechanism and control of pore pressure changes within the landslide is located in the deeper subsurface below or at the lowest parts of the shallow coherent aquifer. Evidence for its existence is mainly based on the estimated recharge areas of water sampled in the wells a few tens of metres below the surface. Inferred long flow distances indicate flow routes transporting water from the uppermost parts of the DSGSD towards the deep-seated parts of the active landslide area. Considering the topographic properties within the DSGSD catchment, this transport mechanism requires slope-discordant flow paths which are at least twice as long as the flow distances estimated for the shallow flow system. These findings allowed us to prepare a conceptual model of groundwater movement within the DSGSD in order to describe identified flow mechanisms along the slope (Fig. 11, Table 3).
Hydrogeological monitoring combined with an automated geostatistical approach provides new insights into the hydrogeological drivers of the deep-seated Vögelsberg landslide. Monitoring of precipitation and groundwater enhanced the understanding of the hydrological control of the landslide. Determined stable isotopic compositions of groundwater and precipitation sampled at different elevations allowed us to determine probable recharge elevations. Combined with an index representing the 3D distances to discharge locations, recharge probability maps were reconstructed. Emphasis was placed on accurately estimating the local δ18O gradient of precipitation with respect to the sensitivity of the subsequent estimation of mean recharge elevation. It was found that the isotopic composition of locally sampled precipitation is in agreement with respective time series provided by ANIP. This strengthens the quality of the monitored data and the chosen monitoring approach and justifies the use of the long-term ANIP data for a more robust derivation of probable recharge elevations. Identified altitude gradients of 0.14 ‰, 0.16 ‰, and 0.21 ‰ per 100 m are in the range of gradients in mountain regions around the globe (0.15 ‰–0.5 ‰ per 100 m; Clark and Fritz, 1997) and in good agreement with the Austrian mean gradient of 0.16 ‰ per 100 m (Humer et al., 1995). Furthermore, these gradients are in good agreement with a value of 0.18 ‰ per 100 m identified by Madritsch and Millen (2007) in a study approximately 5 km south of the Vögelsberg landslide. From this we propose an enlarged area of applicability for the gradient approach used in the present study. The confidence interval of the gradients should therefore be valid at least within the area between the Vögelsberg study area, the Innsbruck and Patscherkofel ANIP stations, and the study area of Madritsch and Millen (2007).
The low temporal variability in the isotopic composition of the groundwater indicates significant attenuation of the seasonal δ18O signal by mixing and long transit times which are expressed by low ITTPs observed within the aquifer. These results justify the usage of multi-annual δ18O ratios of precipitation to derive altitude gradients in order to estimate the mean recharge elevations of the springs. Although low ITTPs obtained in this study indicate transit times of up to 3.5 years (Tetzlaff et al., 2009), our previous study (Pfeiffer et al., 2021) has shown that the landslide responds to hydro-meteorological events with a delay of up to 50 d. This distinct disparity between response time and transit time indicates that the propagation of pore pressure within the aquifer is multiple times faster than the subsurface flow itself. Although the calculation of ITTP was not feasible for the well samples (KB1 and KB2) due to too few multi-temporal measurements, the constant δ18O ratios between single measurement campaigns are interpreted as indicators for comparably long transit times.
Recharge probability maps indicate that groundwater recharges preferably at elevations between 1000 and 1650 m a.s.l. for springs emerging between 800 and 1350 m a.s.l. Water encountered in the wells recharges up to 2200 m a.s.l. The resulting recharge probability maps generally agree with field observations. Areas with high recharge probability are in agreement with mapped areas of geomorphological features supporting groundwater recharge, including open fractures, fissures, up-facing scarps, double-crested ridges, and a generally dry appearance of the humped terrain.
The hydrogeological characteristics of the study area and the low temporal δ18O variability in the groundwater indicate a well-mixed aquifer. It is therefore assumed that the partially reworked and heavily fractured quartz phyllite supports isotropic flow directions. Such conditions are a prerequisite for using the proposed distance-weighted index for differentiating between high and low recharge probabilities at similar elevations. Therefore, we see a high potential for applying our approach to groundwater systems of similar conditions. In the case of mainly anisotropic conditions (e.g. due to one or more prevailing geologic structures), the orientation of the anisotropy controlling the subsurface flow direction must be considered in the assessment of probable recharge areas.
Regarding the comparably higher recharge elevations of groundwater encountered in wells, flow paths following slope-discordant directions had to be introduced to explain groundwater transport, i.e. water flow along the basal shear zone representing a large-scale inhomogeneity at the lower boundary of the fractured and partially disaggregated quartz phyllite. A similar flow mechanism was proposed by Madritsch and Millen (2007) for a DSGSD on the opposite side of the valley. Intact quartz phyllite below the deformed layer is assumed to host almost impermeable hydrogeological conditions. Within the deformed layer slope-discordant groundwater flow is not excluded. Supported by tracer tests, Vallet et al. (2015) and Ronchetti et al. (2020) identified similar slope or slide direction-discordant groundwater flow mechanisms at deep-seated landslides.
The concordance of discharge time series with continuous time series of groundwater level, landslide displacement rate, and precipitation time series indicates that the chosen monitoring interval is sufficient to capture the hydrodynamic behaviour of the respective aquifer in time (Fig. 6). Furthermore, correlations of discharge and landslide velocity indicate that the aquifer controls the landslide's activity. Evidenced by temporal dynamics in the aquifer (spring and piezometer), the three landslide-triggering hydro-meteorological events observed and analysed by Pfeiffer et al. (2021) between 2016 and 2019 are extended by three additional landslide acceleration events (summer 2019, late winter/spring 2020, and late summer/autumn 2020) (Fig. 6). Differences in the temporal variability in discharge among springs in the study area show an elevation-staggered behaviour. Discharge of springs at lower elevations increases earlier than the discharge at higher-elevation springs. This behaviour was characteristic for the snowmelt season in 2019 when for the same time span a fast landslide response to a distinct snowmelt event at elevations below 1700 m a.s.l. was observed (Pfeiffer et al., 2021). Results of the present study suggest that this landslide acceleration event was triggered by recharge of the shallow parts of the aquifer right above the active landslide. Snowmelt started earlier at lower elevations (approx. 1000 m a.s.l.) and successively moved to elevations of up to 1600 m a.s.l. Infiltration due to snowmelt is assumed to be faster than for rainfall, and a concomitant pore pressure increase induces an almost immediate acceleration of the landslide movement.
In addition to this proximal, shallow, and topographically controlled flow system, recharge elevations of the deeper groundwater indicate a more distal deep-seated flow system which transports water originating in the uppermost areas of the DSGSD catchment. Rainfall-induced landslide acceleration as observed by Pfeiffer et al. (2021) in 2016 and 2017 is assumed to be the response to both recharge of the proximal flow system and recharge and pore pressure rise caused by the distal flow system. The longer response time of 24 to 50 d estimated for these events therefore is the consequence of the longer distance of up to 3000 m between recharge area and landslide. With the available data we could not identify if there is distinct mixing between the deeper distal flow and the shallow proximal flow system. Nevertheless, we do not exclude a vertical exchange between shallow and deeper water. It is likely that the distal flow system occurs below the proximal flow system and along the lower boundary of the deformed rock mass.
Similar hydrogeological mechanisms were described by Guglielmi et al. (2002) at the La Clapière and Séchilienne landslides, where also a shallow and a deep groundwater flow system were proposed. Comparable to our study, Vallet et al. (2015) interpreted potential recharge elevations for the Séchilienne landslide. They used the estimated recharge elevation and its intersection along the slope line or on a slope-discordant line reaching higher topographic elevations to distinguish between topographically controlled and structurally controlled subsurface flow paths. The maps of probable recharge areas for the Vögelsberg landslide show comparable results. Thus, our method provides objective information for planning mitigation measures to drain unstable slopes. On the other hand, future drainage systems aiming at reducing groundwater recharge could modify the δ18O composition of springs and hence the recharge probability maps. In this way, the impact of the mitigation strategy could be evaluated and subsequently improved.
This study presents a new approach for assessing groundwater recharge areas to improve the understanding of the hydrogeology of slopes. The highly automated geo-statistical approach yields recharge probability maps based on stable isotope data and a digital elevation model (DEM). It was applied to the Vögelsberg landslide, a currently active slab of a DSGSD in the Watten valley (Tyrol, Austria). Local δ18O altitude gradients of precipitation are in agreement with gradients derived from long-term measurements at stations of the ANIP network. The established local δ18O altitude gradient allowed us to estimate the mean recharge elevation of groundwater sampled at springs and in two wells. The recharge areas were then further constrained based on 3D distances to the spring locations, computed with the help of a DEM.
The resulting recharge probability maps suggest a dual hydrogeological control: a proximal control provided by a shallow aquifer recharging between 1000 and 1650 m a.s.l. and distal flow of groundwater originating in the uppermost areas of the DSGSD. Both the distal and the proximal flow mechanisms are compared and are in accordance with the previously identified hydro-meteorological triggering of landslide acceleration events at this site (Pfeiffer et al., 2021).
The study illustrates the strength of stable water isotopes as a natural tracer to identify and constrain groundwater flow paths. These data helped us to fully understand the hydrogeological controls of the Vögelsberg landslide. Furthermore, the recharge probability maps provide valuable information for mitigation measures (e.g. drainage systems).
Code is available from the authors upon request.
The hydrogeological data that support the findings of this study are openly available in the Zenodo repository at https://doi.org/10.5281/zenodo.5817141 (Pfeiffer et al., 2022).
JP, TZ, and JS developed the research concept, acquired necessary field data, and carried out the analysis. JP drafted the manuscript. TZ, JS, TB, MR, and CS reviewed the manuscript, and all authors contributed to the editing and revision process.
The contact author has declared that none of the authors has any competing interests.
Publisher's note: Copernicus Publications remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
We thank the Federal State of Tyrol (“Abteilung Geoinformation” and “Landesgeologie”) and the Austrian Service for Torrent and Avalanche Control for providing data and a valuable exchange of information. We thank Tim Phillip for analysing the water samples and Sebastian Gehring for assistance in the field.
The present study was conducted within the OPERANDUM project. This project has received funding from the European Union's Horizon 2020 research and innovation programme under grant agreement no. 776848.
This paper was edited by Andreas Günther and reviewed by Catherine Bertrand and one anonymous referee.
Binet, S., Jomard, H., Lebourg, T., Guglielmi, Y., Tric, E., Bertrand, C., and Mudry, J.: Experimental analysis of groundwater flow through a landslide slip surface using natural and artificial water chemical tracers, Hydrol. Process., 21, 3463–3472, https://doi.org/10.1002/hyp.6579, 2007. a, b
Blasch, K. W. and Bryson, J. R.: Distinguishing Sources of Ground Water Recharge by Using δ2H and δ18O, Groundwater, 45, 294–308, https://doi.org/10.1111/j.1745-6584.2006.00289.x, 2007. a, b
Bogaard, T., Guglielmi, Y., Marc, V., Emblanch, C., Bertrand, C., and Mudry, J.: Hydrogeochemistry in landslide research: a review, Bulletin de la Société Géologique de France, 178, 113–126, https://doi.org/10.2113/gssgfbull.178.2.113, 2007. a
Bogaard, T. A. and Greco, R.: Landslide hydrology: from hydrology to pore pressure, WIREs Water, 3, 439–459, https://doi.org/10.1002/wat2.1126, 2016. a
Bonzanigo, L., Eberhardt, E., and Loew, S.: Long-term investigation of a deep-seated creeping landslide in crystalline rock. Part I. Geological and hydromechanical factors controlling the Campo Vallemaggia landslide, Can. Geotech. J., 44, 1157–1180, https://doi.org/10.1139/T07-043, 2007. a
Bouchaou, L., Michelot, J., Vengosh, A., Hsissou, Y., Qurtobi, M., Gaye, C., Bullen, T., and Zuppi, G.: Application of multiple isotopic and geochemical tracers for investigation of recharge, salinization, and residence time of water in the Souss–Massa aquifer, southwest of Morocco, J. Hydrol., 352, 267–287, https://doi.org/10.1016/j.jhydrol.2008.01.022, 2008. a
Cappa, F., Guglielmi, Y., Soukatchoff, V., Mudry, J., Bertrand, C., and Charmoille, A.: Hydromechanical modeling of a large moving rock slope inferred from slope levelling coupled to spring long-term hydrochemical monitoring: example of the La Clapière landslide (Southern Alps, France), J. Hydrol., 291, 67–90, https://doi.org/10.1016/j.jhydrol.2003.12.013, 2004. a, b
Cervi, F., Ronchetti, F., Martinelli, G., Bogaard, T. A., and Corsini, A.: Origin and assessment of deep groundwater inflow in the Ca' Lita landslide using hydrochemistry and in situ monitoring, Hydrol. Earth Syst. Sci., 16, 4205–4221, https://doi.org/10.5194/hess-16-4205-2012, 2012. a, b
Chalikakis, K., Plagnes, V., Guerin, R., Valois, R., and Bosch, F. P.: Contribution of geophysical methods to karst-system exploration: an overview, Hydrogeol. J., 19, 1169, https://doi.org/10.1007/s10040-011-0746-x, 2011. a
Cignetti, M., Godone, D., Zucca, F., Bertolo, D., and Giordan, D.: Impact of Deep-seated Gravitational Slope Deformation on urban areas and large infrastructures in the Italian Western Alps, Sci. Total Environ., 740, 140360, https://doi.org/10.1016/j.scitotenv.2020.140360, 2020. a
Clark, I. D. and Fritz, P.: Environmental isotopes in hydrogeology, 1st Edn., CRC Press, https://doi.org/10.1201/9781482242911, 1997 a, b
Cronshey, R., McCuen, R. H., Miller, N., Rawls, W., Robbins, S., and Woodward, D.: Urban hydrology for small watersheds, US Dept. of Agriculture, Soil Conservation Service, Engineering Division, https://tamug-ir.tdl.org/handle/1969.3/24438 (last access: 28 June 2022), 1986. a
Crosta, G., Frattini, P., and Agliardi, F.: Deep seated gravitational slope deformations in the European Alps, Tectonophysics, 605, 13–33, https://doi.org/10.1016/j.tecto.2013.04.028, 2013. a
Dansgaard, W.: Stable isotopes in precipitation, Tellus, 16, 436–468, https://doi.org/10.3402/tellusa.v16i4.8993, 1964. a
Eberhardt, E., Bonzanigo, L., and Loew, S.: Long-term investigation of a deep-seated creeping landslide in crystalline rock. Part II. Mitigation measures and numerical modelling of deep drainage at Campo Vallemaggia, Can. Geotech. J., 44, 1181–1199, https://doi.org/10.1139/T07-044, 2007. a
Gröning, M., Lutz, H., Roller-Lutz, Z., Kralik, M., Gourcy, L., and Pöltenstein, L.: A simple rain collector preventing water re-evaporation dedicated for δ18O and δ2H analysis of cumulative precipitation samples, J. Hydrol., 448–449, 195–200, https://doi.org/10.1016/j.jhydrol.2012.04.041, 2012. a
Guglielmi, Y., Vengeon, J., Bertrand, C., Mudry, J., Follacci, J., and Giraud, A.: Hydrogeochemistry: an investigation tool to evaluate infiltration into large moving rock masses (case study of La Clapière and Séchilienne alpine landslides), Bull. Eng. Geol. Environ., 61, 311–324, https://doi.org/10.1007/s10064-001-0144-z, 2002. a, b, c, d, e, f
Hilberg, S.: Review: Natural tracers in fractured hard-rock aquifers in the Austrian part of the Eastern Alps–previous approaches and future perspectives for hydrogeology in mountain regions, Hydrogeol. J., 24, 1091–1105, https://doi.org/10.1007/s10040-016-1395-x, 2016. a
Hilberg, S. and Riepler, F.: Interaction of various flow systems in small alpine catchments: conceptual model of the upper Gurk Valley aquifer, Carinthia, Austria, Hydrogeol. J., 24, 1231–1244, https://doi.org/10.1007/s10040-016-1396-9, 2016. a, b
Hofmann, R. and Sausgruber, J. T.: Creep behaviour and remediation concept for a deep-seated landslide, Navistal, Tyrol, Austria, Geomech. Tunnel., 10, 59–73, https://doi.org/10.1002/geot.201600066, 2017. a
Humer, G., Herlicska, H., Rank, D., Trimborn, P., and Stichler, W.: Niederschlagsisotopenmessnetz Österreich, Bundesministerium für Umwelt, ISBN 3-85457-223-9, 1995. a
Jasechko, S., Birks, S. J., Gleeson, T., Wada, Y., Fawcett, P. J., Sharp, Z. D., McDonnell, J. J., and Welker, J. M.: The pronounced seasonality of global groundwater recharge, Water Resour. Res., 50, 8845–8867, https://doi.org/10.1002/2014WR015809, 2014. a
Jomard, H., Lebourg, T., and Tric, E.: Identification of the gravitational boundary in weathered gneiss by geophysical survey: La Clapière landslide (France), J. Appl. Geophys., 62, 47–57, https://doi.org/10.1016/j.jappgeo.2006.07.003, 2007. a
Koltai, G., Ostermann, M., Cheng, H., and Spötl, C.: Can vein-filling speleothems constrain the timing of deep-seated gravitational slope deformation? A case study from the Vinschgau (Italian Alps), Landslides, 15, 2243–2254, https://doi.org/10.1007/s10346-018-1032-y, 2018. a
Kumar, P., Debele, S. E., Sahani, J., Rawat, N., Marti-Cardona, B., Alfieri, S. M., Basu, B., Basu, A. S., Bowyer, P., Charizopoulos, N., Gallotti, G., Jaakko, J., Leo, L. S., Loupis, M., Menenti, M., Mickovski, S. B., Mun, S.-J., Gonzalez-Ollauri, A., Pfeiffer, J., Pilla, F., Pröll, J., Rutzinger, M., Santo, M. A., Sannigrahi, S., Spyrou, C., Tuomenvirta, H., and Zieher, T.: Nature-based solutions efficiency evaluation against natural hazards: Modelling methods, advantages and limitations, Sci. Total Environ., 784, 147058, https://doi.org/10.1016/j.scitotenv.2021.147058, 2021a. a
Kumar, P., Debele, S. E., Sahani, J., Rawat, N., Marti-Cardona, B., Alfieri, S. M., Basu, B., Basu, A. S., Bowyer, P., Charizopoulos, N., Jaakko, J., Loupis, M., Menenti, M., Mickovski, S. B., Pfeiffer, J., Pilla, F., Pröll, J., Pulvirenti, B., Rutzinger, M., Sannigrahi, S., Spyrou, C., Tuomenvirta, H., Vojinovic, Z., and Zieher, T.: An overview of monitoring methods for assessing the performance of nature-based solutions against natural hazards, Earth-Sci. Rev., 217, 103603, https://doi.org/10.1016/j.earscirev.2021.103603, 2021b. a
Lacroix, P., Handwerger, A. L., and Bièvre, G.: Life and death of slow-moving landslides, Nat. Rev. Earth Environ., 1, 404–419, https://doi.org/10.1038/s43017-020-0072-8, 2020. a
Lajaunie, M., Gance, J., Nevers, P., Malet, J.-P., Bertrand, C., Garin, T., and Ferhat, G.: Structure of the Séchilienne unstable slope from large-scale three-dimensional electrical tomography using a Resistivity Distributed Automated System (R-DAS), Geophys. J. Int., 219, 129–147, https://doi.org/10.1093/gji/ggz259, 2019. a
Liebminger, A., Haberhauer, G., Papesch, W., and Heiss, G.: Correlation of the isotopic composition in precipitation with local conditions in alpine regions, J. Geophys. Res.-Atmos., 111, D05104, https://doi.org/10.1029/2005JD006258, 2006. a, b, c
Madritsch, H. and Millen, B. M. J.: Hydrogeologic evidence for a continuous basal shear zone within a deep-seated gravitational slope deformation (Eastern Alps, Tyrol, Austria), Landslides, 4, 149–162, https://doi.org/10.1007/s10346-006-0072-x, 2007. a, b, c, d, e, f, g
McGuire, K. J., McDonnell, J. J., Weiler, M., Kendall, C., McGlynn, B. L., Welker, J. M., and Seibert, J.: The role of topography on catchment-scale water residence time, Water Resour. Res., 41, W05002, https://doi.org/10.1029/2004WR003657, 2005. a
Mikoš, M., Četina, M., and Brilly, M.: Hydrologic conditions responsible for triggering the Stože landslide, Slovenia, Eng. Geol., 73, 193–213, https://doi.org/10.1016/j.enggeo.2004.01.011, 2004. a
Montety, V. d., Marc, V., Emblanch, C., Malet, J.-P., Bertrand, C., Maquaire, O., and Bogaard, T.: Identifying the origin of groundwater and flow processes in complex landslides affecting black marls: insights from a hydrochemical survey, Earth Surf. Proc. Land., 32, 32–48, https://doi.org/10.1002/esp.1370, 2007. a
Mook, W.: Introduction to Isotope Hydrology. Stable and Radioactive Isotopes of Hydrogen, Oxygen and Carbon., Taylo & Francis Group, ISBN 9780415398053, 2006. a
Moser, H. and Rauert, W.: Lehrbuch der Hydrogeologie-Isotopenmethoden in der Hydrologie, Gebr. Bornträger, Berlin, Stuttgart, ISBN 978-3-443-01012-6, 1980. a, b
Pfeiffer, J., Zieher, T., Schmieder, J., Rutzinger, M., and Strasser, U.: Spatio-temporal assessment of the hydrological drivers of an active deep-seated gravitational slope deformation: The Vögelsberg landslide in Tyrol (Austria), Earth Surf. Proc. Land., 46, 1865–1881, https://doi.org/10.1002/esp.5129, 2021. a, b, c, d, e, f, g, h, i, j, k
Pfeiffer, J, Zieher, T., Schmieder, J., Bogaard, T., Rutzinger, M., and Spötl, C.: Hydrogeological data of groundwater and precipitation monitored in the Vögelsberg landslide catchment (1.0), Zenodo [data set], https://doi.org/10.5281/zenodo.5817141, 2022. a
R Core Team: R: A Language and Environment for Statistical Computing, R Foundation for Statistical Computing, Vienna, Austria, https://www.R-project.org/, last access: 30 November 2021. a
Rockenschaub, M., Kolenprat, B., and Nowotny, A.: Innsbrucker Quarzphyllitkomplex, Tarntaler Mesozoikum, Patscherkofelkristallin, Geologische Bundesanstalt – Arbeitstagung 2003: Blatt 148 Brenner, 41–58, https://opac.geologie.ac.at/wwwopacx/wwwopac.ashx?command=getcontent&server=images&value=ATA_2003_Seite_001_282.pdf (last access: 28 June 2021), 2003. a
Rodgers, P., Soulsby, C., and Waldron, S.: Stable isotope tracers as diagnostic tools in upscaling flow path understanding and residence time estimates in a mountainous mesoscale catchment, Hydrol. Process., 19, 2291–2307, https://doi.org/10.1002/hyp.5677, 2005a. a
Rodgers, P., Soulsby, C., Waldron, S., and Tetzlaff, D.: Using stable isotope tracers to assess hydrological flow paths, residence times and landscape influences in a nested mesoscale catchment, Hydrol. Earth Syst. Sci., 9, 139–155, https://doi.org/10.5194/hess-9-139-2005, 2005b. a
Ronchetti, F., Piccinini, L., Deiana, M., Ciccarese, G., Vincenzi, V., Aguzzoli, A., Malavasi, G., Fabbri, P., and Corsini, A.: Tracer test to assess flow and transport parameters of an earth slide: The Montecagno landslide case study (Italy), Eng. Geol., 275, 105749, https://doi.org/10.1016/j.enggeo.2020.105749, 2020. a, b, c, d
Scanlon, B. R., Healy, R. W., and Cook, P. G.: Choosing appropriate techniques for quantifying groundwater recharge, Hydrogeol. J., 10, 18–39, https://doi.org/10.1007/s10040-001-0176-2, 2002. a
Schmieder, J., Hanzer, F., Marke, T., Garvelmann, J., Warscher, M., Kunstmann, H., and Strasser, U.: The importance of snowmelt spatiotemporal variability for isotope-based hydrograph separation in a high-elevation catchment, Hydrol. Earth Syst. Sci., 20, 5015–5033, https://doi.org/10.5194/hess-20-5015-2016, 2016. a
Siemon, B., Christiansen, A. V., and Auken, E.: A review of helicopter-borne electromagnetic methods for groundwater exploration, Near Surf. Geophys., 7, 629–646, https://doi.org/10.3997/1873-0604.2009043, 2009. a
Strauhal, T., Prager, C., Millen, B., Spoetl, C., Zangerl, C., and Brandner, R.: Aquifer geochemistry of crystalline rocks and Quaternary deposits in a high altitude alpine environment (Kauner Valley, Austria), Aust. J. Earth Sci., 109, 29–44, https://doi.org/10.17738/ajes.2016.0002, 2016. a
Tetzlaff, D., Soulsby, C., Waldron, S., Malcolm, I. A., Bacon, P. J., Dunn, S. M., Lilly, A., and Youngson, A. F.: Conceptualization of runoff processes using a geographical information system and tracers in a nested mesoscale catchment, Hydrol. Process., 21, 1289–1307, https://doi.org/10.1002/hyp.6309, 2007. a
Tetzlaff, D., Seibert, J., McGuire, K. J., Laudon, H., Burns, D. A., Dunn, S. M., and Soulsby, C.: How does landscape structure influence catchment transit time across different geomorphic provinces?, Hydrol. Process., 23, 945–953, https://doi.org/10.1002/hyp.7240, 2009. a, b, c, d, e
Vallet, A., Bertrand, C., Mudry, J., Bogaard, T., Fabbri, O., Baudement, C., and Régent, B.: Contribution of time-related environmental tracing combined with tracer tests for characterization of a groundwater conceptual model: a case study at the Séchilienne landslide, western Alps (France), Hydrogeol. J., 23, 1761–1779, 2015. a, b, c, d, e, f
van Geldern, R. and Barth, J. A.: Optimization of instrument setup and post-run corrections for oxygen and hydrogen stable isotope measurements of water by isotope ratio infrared spectroscopy (IRIS), Limnol. Oceanogr.: Meth., 10, 1024–1036, https://doi.org/10.4319/lom.2012.10.1024, 2012. a
Welch, L. A. and Allen, D. M.: Hydraulic conductivity characteristics in mountains and implications for conceptualizing bedrock groundwater flow, Hydrogeol. J., 22, 1003–1026, https://doi.org/10.1007/s10040-014-1121-5, 2014. a
Zieher, T., Markart, G., Ottowitz, D., Römer, A., Rutzinger, M., Meißl, G., and Geitner, C.: Water content dynamics at plot scale – comparison of time-lapse electrical resistivity tomography monitoring and pore pressure modelling, J. Hydrol., 544, 195–209, https://doi.org/10.1016/j.jhydrol.2016.11.019, 2017. a